To most outsiders, modern mathematics is unknown territory. Its borders are protected by dense thickets of technical terms; its landscapes are a mass of indecipherable equations and incomprehensible concepts. Few realize that the world of modern mathematics is rich with vivid images and provocative ideas. -Ivars Peterson
Those of you who know me well know that I have little love for math for its own sake, but that I prize it as an incredible tool to help us understand and quantify physical systems. This has incredible applications, from figuring out what trajectory will succeed in a game of basketball,

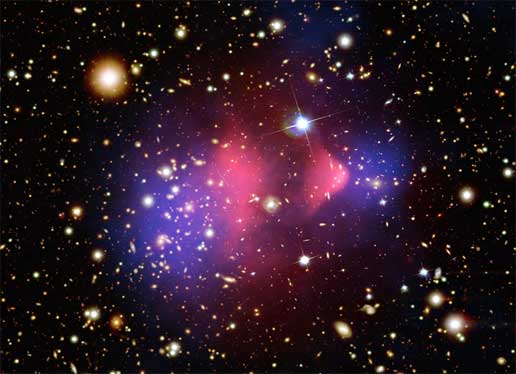
to being able to predict what happens to matter -- both dark matter and normal matter -- when galaxy clusters collide.
Well, I'm teaching my very first advanced undergraduate course this semester, on electricity and magnetism.

To nobody's surprise, it's pretty mathematically intensive, but what's missing from most textbooks and E&M courses are physical explanations of what the mathematics means. For instance, I've started teaching about fields, and pretty much every textbook out there goes on and on about the properties of fields. They say you can do three things to fields, take the gradient, divergence, or curl of them.
(Are you asleep yet? I'm sorry!)
What do these things mean? An easy way to picture it is in terms of water. If you placed a drop of water anywhere on, say, Earth, the magnitude and direction of how it rolls down is the gradient of the Earth's elevation.

If you let that drop of water flow, as it goes downhill, it can either spread out or converge to a narrower stream. When we quantify that, that's what the divergence of the field is.

And finally, when that water is flowing, sometimes it gets an internal rotational motion, like an eddy. A measure of that rotational motion is called the curl of the field.

Well, one math geek statement is as follows: the curl of the gradient of a scalar field is always zero. What does this mean, in terms of our water? It means that I can take any topography I can find, invent, or even dream up.
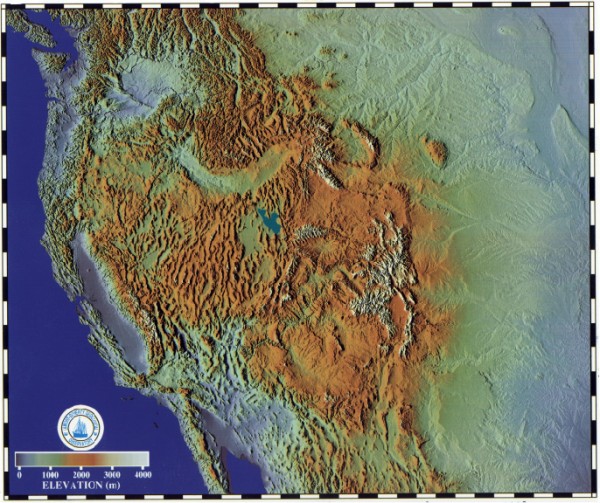
I can drop a tiny droplet of water on it anywhere I like, and while the water may roll downhill (depending on the gradient), and while the water may spread out or narrow (depending on the divergence of the gradient), it will not start to rotate. For rotation to happen, you need something more than just a drop starting out on a hill, no matter how your hill is shaped! That's what it means when someone says, "The curl of the gradient is zero."
So, you know, the next time you're at a bar, and some friendly math geek comes up to you (they're easy to spot by their T-shirts),

you'll actually be able to talk vector calculus with them. Err... maybe that isn't the best idea after all. But you will know what divergence, curl, and gradient mean, and how many people will know that at bar trivia?

It just so happens that this is also my first semester of advanced undergraduate electricity and magnetism. I struggled with these concepts in vector calculus, given no way to visualize what the terms actually 'meant', other than a string of operations on scalar or vector functions. Thank you for these concise analogies, this kind of explanation is so much better for visual thinkers such as myself. I hope I'll get to see more articles like this in the future as the class progresses.
Would you, please, discuss the rotation of water as it goes down a drain (sinkhole)?
Thanks, Neil
Funny, those metaphors about water flow are exactly what math professors use when trying to motivate the operations in a multivariable calculus context. Sounds like physics professors are yet again sloughing off teaching onto mathematics as a "service department" while vacuuming up the lion's share of grant funding.
Eek! E&M! E&M I wasn't too bad. I had a goofy, stereotypical physics prof with a frizzy white fro, who wore the same pair of khakis every day, and insisted we call 1 "the loneliest number." We learned about capacitors with hippopotamuses carrying charges. We had to write a poem for one of the questions on the final.
Perhaps this is why I was woefully unprepared for the horror that is E&M II. Ugh. I love physics, but that class was pure torture.
Never had too many problems with the vector calculus, though. That part is cool!
Honestly, I find the mathematical definitions more intuitive in some weird way than the physical definition...
Thank you Ethen, I enjoyed your analogies, will you please continue with your teachings and explain the curl operator, in relation with E and B, when we dot or cross product the curl with a vector field what happens?
Thanks,
Ash
It's amazing how subtly misleading all of those analogies are.
Wow, where did you get the picture of the woman with the Van De Graf generator? I went to undergrad with her.
The T-shirt doesn't make sense; it must be an engineer's shirt, not a mathematician's.
@Adrian: See if the library has a copy of the calculus volumes by Courant and John. If you can work your way through those books, they're the best calculus books I've ever seen - by a long shot. The authors try to relate the math to the real world and also explain how one thing leads to another rather than just hammering you with relationships. Patience is necessary though since the authors' style often leads to what some might think of as long and unnecessary detours, but the authors are actually showing how things are related and how they developed (from a short, not-as-sloppy-as-reality point of view).
How could anyone *not* like math for math's sake? [shudder]
;^)
I love it and I'm glad that my husband knows what I'm talking about and that if I'm asking a physics question he knows how to answer it. He speaks math too, even though he seems to think that I have a bit of a fetish about it. :^O
Nice explanations. :-) In my physics lectures, we learned it in similar ways - calling grad the "vector of steepest descent", saying that div tells you the sources and sinks (although water wasn't directly mentioned there, IIRC), and rot tells you the "eddies". But the explanation for rot grad = 0 is new to me - great way of stating that! Now, what about a similar nice explanation for div rot = 0? ;-)
http://www.acceleratingfuture.com/michael/blog/images/EliezerBayes.jpg
@9: Multiplication by i rotates the complex plane by 90 degrees, duh!
That's how my physics professors explained it, too. I think my textbooks might have as well...
Boas' Mathematical Methods in Physics is very good for teaching us lowly physics types vector calculus. And Griffiths always does a good job in his E&M and Quantum Mechanics textbooks.
I, too, was rather annoyed at various courses of physics (and occasionally chemistry, to be fair) reïnventing the wheel to explain maths that we'd all learnt the first two semesters.
--o--
I know it's a girl, but the resemblance is striking.
A real mathematician's shirt would have the angle as Pi/2 [rad].
so...where can I get the shirt?
(yes, I'm an engineer....what's your point?)
@themann1086
Griffiths! I don't know what's wrong with me, but I cannot stand the Griffiths texts. Like I said, E&M II was a nightmare, and I think in large part due to that awful textbook. The quantum one was better, but still just not very helpful for me.
One of my professors (Tankersley, known simply as "Tank") who was older than old and way too smart, wrote his own "handouts," which compiled into a textbook, and they were way better for me for the math related to quantum mechanics.
Looks to me like you've just made two contradictory statements:
"If you placed a drop of water anywhere on, say, Earth ... and finally, when that water is flowing, sometimes it gets an internal rotational motion, like an eddy. A measure of that rotational motion is called the curl of the field. "
"I can take any topography I can find, invent, or even dream up ... I can drop a tiny droplet of water on it anywhere I like ... it will not start to rotate"
Well, will it or won't it? Of course, it won't so your initial analogy for curl probably needs to talk about the other conditions needed for rotational motion.
Or am I missing something?
(Incidentally, thanks for the dark matter series and the new big bang series!)
I see a subtle flaw in the analogy: the water only flows on part of the surface, so water flowing downhill could have a net rotation (even though the integrated curl over the surface is zero). Consider an old music record. Make it a cone by lifting its center while keeping the outside rim fixed. Now put a tiny drop of water in a groove. By the time it reaches bottom, it will have a net rotation (integral around the rim of the tangent part of the water velocity). But the integral of the curl of the field over the surface is zero becuase it includes not just the grooves but also the ridges.
By the way, I hated Jackson. Thoroughly boring book. Take a look at Principles of Electrodynamics by Schwartz. $12 from Dover, won't help you decompose plane waves in cylindrical coordinates and match border conditions to an elliptical object but very intuitive and clear.
Any analogy is flawed- if it wasn't, it would be the real thing. The trick is that a good analogy (or metaphor) gives us insight that we wouldn't otherwise have, if at the expense of total explanation. The water analogy works well in this light. Remember: 0.5% of the population are abstract thinkers, 4.5% are concrete thinkers and the remaining 95% of us are reinforced concrete thinkers, so anything that sheds light on the Universe in those terms is useful.
ROFLmeow @ #23! Gotta remember that one! =^..^=
cheers, just thought: isn't the gradient the direction to move in the domain for the fastest increase of function value? if so I'd say that the water would be running "down" in the direction of the negative gradient =)
This is wonderful analogy. I AM LUCKY to read this site. Please explain all the concepts like this