"One mustn't look at the abyss, because there is at the bottom an inexpressible charm which attracts us." -Gustave Flaubert
The deepest depths of space, out beyond our atmosphere, our Solar System, and even our galaxy, hold the richness of the great Universe beyond. Stretching for billions of light years in every direction, there are structures large and small, dense and sparse, everywhere we've ever dared to look.
Image credit: R. Jay GaBany, Cosmotography.com.
In addition to the visible, luminous matter we see in the image above, there's both non-luminous normal matter and dark matter. The non-luminous matter is made out of protons, neutrons, and electrons, but doesn't emit light. This includes things such as gas, dust, planets, and astrophysicists: in other words, most normal matter in the Universe. But when we take everything we know about normal matter, including how much there is of it, how its pulls together under the force of gravity throughout the Universe, we find that there needs to be about five times as much dark matter as all the normal matter combined.
One of the easiest ways to figure this out and measure it is by looking at some chance locations in the Universe where there are two massive structures directly lined up, one-behind-the-other, relative to our line-of-sight.
Image credit: ESA, NASA, K. Sharon and E. Ofek.
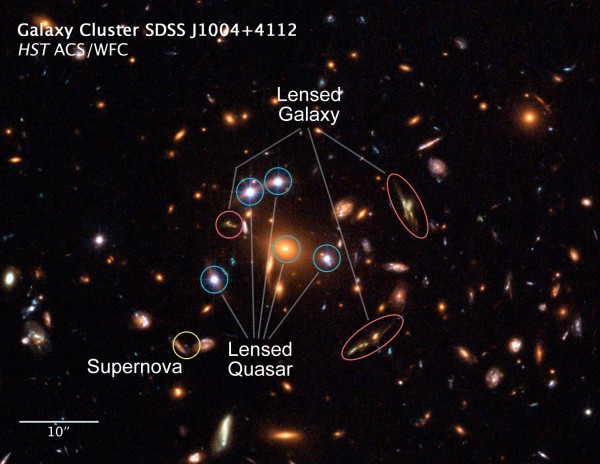
Above is what happens when you have a galaxy cluster with both a quasar and a background galaxy directly behind it. This result -- of multiple images and/or distorted, arcing appearances of the background source(s) -- comes about because of gravitational lensing. This intervening mass bends and magnifies the light from the background source, allowing us to see incredibly distant objects that would be otherwise invisible.
It works the other way, too. From the light that we observe from these background objects, we can infer all sorts of things -- like the mass and how it's distributed -- of the intervening, foreground object, as well as how perfectly/imperfectly it's aligned with the background ones.
The results are often breathtaking and, at least to me, always spectacular.
Image credit: ESA/Hubble & NASA, retrieved from APOD.
The "horseshoe" above represents a nearly perfect alignment of two sources. Almost perfect, but not quite. If the background and foreground sources were perfectly aligned, the background source would be bend into a uniform-brightness circle -- a great cosmic rarity -- known as an Einstein ring.
If you could zoom into a nearly-but-not-quite-perfect Einstein ring that was lensed by a black hole, the sight would surely, for as long as you remained an intact being, blow your mind. For what you'd find would be an infinite sequence of these rings, progressively decreasing in brightness, as you approached the event horizon.
Image credit: Public Domain image, retrieved from Wikipedia.
But I digress. These Einstein rings are lensed by galaxies, not black holes. The circles they make are never exactly perfect, but some come close. In particular, here's a (falsely-colored) image of one that was recently discovered. This one is particularly interesting for the sheer distances involved: the foreground galaxy -- the one doing the lensing -- is a luminous red galaxy located 9.8 billion light years away. But the background galaxy, the one bent into the ring, is an even more spectacular 17.3 billion light years away. And as you'll notice, it forms a nearly, but not quite, perfect ring.
Image credit: S. Vegetti et al., retrieved from space.com.
This system, known as JVAS B1938+666, is much more than just a pretty ring of nearly-perfectly aligned galaxies. You remember, when you form a ring like this, one of the things you'll learn is how the foreground mass is distributed. In addition to the central, luminous red galaxy, there's also a dark concentration of mass, a bit off from the center, of about two hundred million Suns.
Incorrectly reported by many as a purely dark matter galaxy, this is simply a standard dwarf galaxy, but it's so far away that the light from its stars are insufficiently bright to be seen, even with a ten-meter telescope!
Image credit: David Lagattuta / W. M. Keck Observatory.
So what's really going on here? You can take a look at the full paper (S. Vegetti et al., 2012) for yourself, but let's break it down in simple terms, and (hopefully) clear up the confusion surrounding this. Almost everywhere in the Universe, the structure you form is about 80-85% dark matter and 15-20% normal matter.
Everywhere. In our galaxy, in galaxy clusters, even in superclusters on the largest visible scales. In these large objects, the gravitational forces are huge, and the gas, dust, and all the forms of normal matter stay bound to their parent object, no matter what you do to it.
Image credit: NASA, ESA, and A. Aloisi (ESA & STScI).
But in dwarf galaxies, the little guys, large bursts of star formation can be so powerful that they can eject normal matter out of the galaxy itself! This allows them to, over time, become galaxies that are even more dark-matter-dominated than other, more massive objects in the Universe. In the image above, I Zwicky 18 is full of young stars, indicating an intense burst of star formation that's no more than 500 million years old. There are older stars in there, too, which are more like 10 billion years old, but this latest burst, as perhaps the image below shows even more clearly, will turn this galaxy into an even more strongly dark-matter-dominated object in the future.
Image credit: Centro de Astrofísica da Universidade do Porto, retrieved from sciencedaily.com.
As this intense burst of star formation happens, bright, hot, young stars form, burn brightly, and die in spectacular supernova explosions. The radiation and outward flux from these objects can heat up and energize the normal, non-luminous matter so thoroughly that it can achieve escape velocity, kicking it out of the dwarf galaxy! What gets left behind are the relic, long-lived stars, the old stellar corpses, and the dark matter.
But what's really important here is that this is exactly what we know should happen! Despite what you may have read elsewhere, there's no reason to believe these objects are 100% dark matter, and there's definitely no reason to believe these observations pose a problem for structure formation. Some people argue that dark matter simulations predict a different density of dwarf galaxies than our Local Group has, and therefore dark matter is wrong. But we have to look at the entire Universe! This one fact does not mean that our Local Group is a good representation of the rest of the Universe; in fact we already know many ways in which it is definitely not! So we go to the paper itself, wherein they've found the most distant dwarf galaxy ever, which says:
Our results are consistent with the predictions from cold dark matter simulations at the 95 per cent confidence level, and therefore agree with the view that galaxies formed hierarchically in a Universe composed of cold dark matter.
And this is as close to a definite (12-σ significance!) detection as one could get: see for yourself!
Image credit: S. Vegetti et al., Figure 3 in the pdf.
You're always going to make waves by claiming that you've done something sensational, like disproven dark matter or found an object made out of 100% dark matter and 0% normal matter, but that's not what we have here.
We've just discovered, at nearly 10 billion light years away, the most distant low-mass, dwarf galaxy in the Universe. And that should be impressive enough; do you know how hard these things are to find?
Image credit: NASA, ESA, and The Hubble Heritage Team (STScI/AURA).
The closest galaxy to us, the Sagittarius Dwarf Galaxy, is about the same size: 1-200 million solar masses, and it took the freakin' Hubble Space Telescope to take this picture of it! Considering it's only seventy thousand light years away, maybe we can cut ourselves some slack for not being able to see the starlight from its twin ten billion light years away!
And despite the great cosmic distance, we are still able to find it, all thanks to gravitational lensing. Are you not impressed?
- Log in to post comments
17.3 billion light years away? How is it possible for the light to have reached us then, lensed or otherwise? My poor monkey brain! Ethan, please explain.
The universe is expanding while the light is traveling, Brian.
My question is this.. if it requires 5x as much dark matter as normal to account for large scale observations, how come we haven't noticed even the most minute fraction of that in the solar system? Not the orbits of the planets, not even in the age of the sun (isn't the age of a star determined by the mass)? Not even a fraction of a percent?
Brian @1, this article from last year might help you: http://scienceblogs.com/startswithabang/2011/01/q_a_how_is_the_universe…
Jeffrey @2, it's because of how normal matter "clumps" together into things like stars, planets, and galactic disks, while dark matter doesn't. Normal matter can collide with other normal matter, but dark matter neither collides with itself nor with normal matter, so it remains much more diffuse. If you calculated the density of dark matter at our location in the galaxy, and asked how much total mass is there due to dark matter if you drew a big sphere the size of Pluto's orbit centered on the Sun, you'd get something like the mass of Mount Everest. If you can come up with a way to detect that, you'll be a scientific hero.
So dark matter is not really proportionate to normal matter? Some galaxies and solar systems would have massively more and others like ours would have minutely less? I would think if it is so dispersive, it would be more evenly spread out, and follow where normal matter was (and vice versa, gravitationally speaking).
Ethan @3: Could you expand on why dark matter doesn't "clump"? Clearly dark matter does interact gravitationally, so it should be pulled together into denser regions, and matter-dense regions like stars should presumably pull in a halo of dark matter in much the same way that they pulled in interstellar gas during star formation. You mention 'colliding' as being important: is it that non-gravitational interaction (e.g. formation of atoms and molecules via strong and electromagnetic forces) is what enables matter to achieve the much denser "clumping" that 'normal' matter achieves in planets and stars?
Jeffrey @4 and Ivan @5, I do have answers for the questions you're asking, but they would take far too long an answer for a comment here. Perhaps if I can get to it I'll put together a post on this for Friday. Thanks for your questions!
For a more in-depth discussion of Vegetti's paper on JVAS B1938+666, see https://scienceandreason3.wordpress.com/2012/02/27/the-dwarf-satellite-…
It's worth noting that only one single dwarf galaxy was detected, when there could be thousands of such around JVAS B1938+666. The one that was detected is special in being located in just the right position to affect the Einstein ring.
The conclusion that there must be many more has good justification, but it involves some fancy analysis for support.
Thanks Ethan. This lack of local evidence has been my biggest problem with dark matter. It is like, we made all these observations about orbits, and determined how much mass they had to have based on their motions, and then used that to guess the mass of other things, and then saw it didn't work on a large scale, and that doesn't filter back down to why our local observations might be wrong. Wouldn't all sorts of things be affected, like the rotation of stars, the points at when they would nova, or even the period of binary stars.. If we are wrong by a factor of 5-6, then how can we precisely steer probes or sattelites? Aren't some of them making measurements (like the neutrino experiment that is off 60 nanoseconds ) that require incredibly precise knowledge of the mass of the earth and other solar bodies?
@ Ivan
My answer is no substitute for Ethan's, but hey. You are correct that non-gravitational interaction, essentially friction, plays a big role. Clouds of gas and dust collide with each other and itself. If the gas didn't interact with itself, like dark matter, then as the gas collapsed it wouldn't stop and collect in the center it'd keep right on going until it got as far away from the center as it started. Something has to slow the gas down, and it's electromagnetic interaction at close range (friction) that does it, turning the kinetic energy to heat.
This doesn't happen with dark matter. It can pass right through a galaxy without losing significant energy.
Hope that helps.
Jeffrey @8: You are referring to timing measurements that do indeed have to be precise for certain applications (like GPS). But that is because they are trying to measure the difference between 1 and 1 + ε. If, say, ε ~ 10^{-12}, then you need a precision of about one part in 10^{-12} to rule out the possibility of ε = 0. But you do not need anywhere near that precision in your measurement of ε. I'm not sure how well we know the Earth's mass, but we know it well enough to account for GR effects on GPS systems. We probably have larger error bars on the masses of other solar system objects, but we don't need very precise values to calculate the orbits of objects orbiting Earth.
As for dark matter: Keep in mind that, in addition to its low density, the stuff is surrounding us, so the effect of a piece of dark matter in one direction is offset by the effect of a piece of dark matter in the opposite direction. The cancellation is exact if you are at the center of a spherical distribution. We are off center within our galaxy, but the out-of-plane components would tend to cancel, so it would like we are being pulled toward the center of the galaxy by a mass greater than that of the ordinary matter. At the solar system level, the effects would be so small (because you would have to subtract the acceleration of the Sun from that of the Earth or other body) that we can't measure them.
Ethan, while you are at it on the post for Friday, what's the matter on calling dark matter 'dark matter'? I think that people and myself associate properties with DM because of association with (normal) matter but they seem to be completely different beasts. Wouldn't be better to get a different name without 'matter' in it?
When you gaze into the abyss, the abyss gazes right back at you. --Friedrich Nietzsche
hasur @11:
How different is dark matter?
For comparison: photons are different from other physical entities in that photons always move at lightspeed. Arguably photons move according to a law of motion that is unique to photons.
We think of photons as pure Energy. Photons do have inertial mass, as all energy has a corresponding inertial mass, but we don't regard photons as a form of matter, because of the difference.
As far as we can tell dark matter moves in accordance with the universal laws of motion.
In its motion and gravitational interaction dark matter behaves like matter. My guess is that's why it's regarded (and named) as a type of matter.
Ethan very disappointing summary. Usually you are clear even when you hype.
Vegetti's paper is clear, "The gravitational lens system JVAS B1938+666 has a bright infrared background galaxy at redshift 2.059 , which is gravitationally lensed into an almost complete Einstein ring of diameter â¼ 0.9 arcseconds by a massive elliptical galaxy at redshift 0.881" aiXiv papers almost always use z redshift to indicate distance; almost never use the ambiguous "billion of light years" units.
Ethan says, "foreground galaxy -- the one doing the lensing -- is a luminous red galaxy located 9.8 billion light years away. But the background galaxy, the one bent into the ring, is an even more spectacular 17.3 billion light years away." But which "billion light years" definition is Ethan using? The technically correct or the common usage. It all depends on what the definition of is is.
About another object, the New Scientist is clear. "Astronomers have spotted the most distant object yet confirmed in the universe â a self-destructing star that exploded 13.1 billion light years from Earth. It detonated just 630 million years after the big bang, around the end of the cosmic "dark ages", when the first stars and galaxies were lighting up space... This burst lies at a redshift of 8.2" http://www.newscientist.com/article/dn17035-most-distant-object-in-the-…. New Scientists uses the common usage definition where 13.7 billion years ago = 13.7 billion light years away and twice clarifies adding "630 million years after the big bang" and "redshift of 8.2".
How does the "New Scientist's most distant object... 13.1 billion light years" relate to "Ethan's most distant dark galaxy... 17.3 billion light years"? Ethan's object is much closer to Earth.
The most distant object's z = 8.2 means light was emitted 13.1 billion years ago.
Vegetti's z = 2.059 means light was emitted about 10 billion
years ago
Vegetti's z = .881 means that light was emitted about 7 billion years ago
So Ethan mislead (me at least) into thinking that Vegetti's observation was of an object outside the Cosmic event Horizon of 13.7 billion years (beyond which we can see nothing.)And Ethan knew he was being ambiguous.
OK Ethan here is your test,
How many billion light years away is that "New Scientist's most distant object z = 8.2"??
(yes, in the technically correct billion of light year units corresponding to a 94 billion light year radius visible universe.) The relationship is not linear or easily found.
So, Ethan please point to a place on the internet (a chart or a calculator) that quickly converts between
. z = redshift
. billion of years
. billion of light years
I CAN'T FIND ONE.
A 1 page table would be nice.
OKThen,
No one is misleading you. The conversions are easy, well-understood and I make very clear distinctions about whether this is the distance to the object (as I stated here), the distance the object was when the light was emitted (the improper usage I deride when it's used to refer to "distance away") and/or redshift.
You may enjoy Ned Wright's cosmology calculator: http://www.astro.ucla.edu/~wright/CosmoCalc.html
You will have to do your own conversions from pc into ly.
dark matter sounds more and more like phlogiston to me
Ethan
Thanks, Ned's calculator (if I don't cange any of his other 4 variables and just input z) does give the answer. I had looked on Ned's site but did not find the calculator, among a dozen other places. Not easy to find even if looking. Also Ned's calculator doesn't do the reverse calculator; given your distance what is the z or the time?
But I still think this post is overhyped, misleading, confusing and deliberately so; unlike the original aiXiv report and unlike the example quote from New Scientist; neither of which overhype or confuse.
But yes, I am venting frustration.
Ethan
You say "the distance the object was when the light was emitted (the improper usage I deride when it's used to refer to "distance away")"
Please clarify this. Are you saying that the distance where for example numerical distance = numerical time (e.g. 13.1 billion light years means 13.1 billion years ago) means that 13.1 billion lightyears is the distance when the light was emitted. I didn't think that was so. I thought it dependent on what inflation value is choosen. And clearly, a big bang universe was not 13.7 billion light years wide 13.7 billion years ago. It just travelled 13.7 billion years in the interveneing 13.7 billion years plus it travelled the additional inflation distance which as Ned's calculator shows can have various values.
The only two numbers that in my mind can't be derided are z and time.
Finally as I understand it, in a continuously inflating de Sitter universe (which we are presumably); the apparent radius (i.e. distance to the cosmic event horizon) will never change. Meaning an astronomer today sees back 13.7 billion years and an astromomer 100 or a 1,000,000 billion years from yow will see back only 13.7 billion years. Thus z or time as in billions of years ago are two numbers with the least assumptions attached.
OKThen/17:
Here's a list of 4 cosmology calculators, including Ned Wright's: http://ned.ipac.caltech.edu/help/cosmology_calc.html (The NED here is not Ned Wright.)
I haven't tried any except Ned's, since it does what I need (in this context).
To go from distance to z, I use successive approximation. (Make a guess at z and see how close it comes to the distance you wanted.) But there's always the ambiguity of what you mean by "distance". Apparently Ned has "advanced" and "light travel time" versions of his calculator - linked at the page of the basic version. You might want to look through some of his tutorials to clarify what's meant by "distance" - comoving, angular size, luminosity, etc. Which one to use depends on what you're trying to do with it.
Ethan
Sorry. I was frustrated. I should have asked for a calculator link long ago.
You use the correct definition of astronomical distance and I should know that by now. Sometimes I'm a bit daft; but eventually I learn. Thanks.
@19 Charles
Thank you for the links. And thanks for the clarification; I did assume Ned Wright.
Jeffrey, Ivan and others, looks like I'm going to disappoint you and make you wait until monday for the Dark Matter post; too much going on to crank one out in the rest of the day.
Thanks for your patience!
i think its really cool
Hi Ethan,
there is a mistake- you write-
>The closest galaxy to us, the Sagittarius Dwarf Galaxy,...
and the picture to this text show the "Sagittairus dwarf irregular galaxy"- and this galaxy is in a distance of 3,39 ± 0,23 Millionen Lj.
The Sagittarius Dwarf Elliptical Galaxy is the closest galaxy to us with a distance of only 70.000 Lj
There are to different galaxies :)
Stefan