"Arithmetic! Algebra! Geometry! Grandiose trinity! Luminous triangle! Whoever has not known you is without sense!" -Comte de Lautréamont
When you think about it, it's amazing that our physical Universe makes sense at all. The fact that we can observe what's happening, determine the laws that govern it, and predict what will happen under the same or similar circumstances is the most remarkable power that science has. If that's what you're doing in any aspect of your life, congratulations, you are a scientist. But that doesn't tell us, fundamentally, what the Universe is like at its most basic level. Are we made up of point-like particles? Or are they geometric constructions? Are we ripples in the Universe itself? In a way, They Might Be Giants might be pondering exactly this in their song that I present to you this weekend,
At the root of all of this is mathematics, which is in its own way beautiful, elegant, and happens to be our foundation for making sense of the Universe. And in what appeared to be a simple puzzle, I saw an image similar to this one floating around the internet and making the rounds on facebook.
How many triangles are in this image? 92.6% of Americans get this question wrong!
It's pretty straightforward: an equilateral triangle with three extra lines coming out of two of the vertices, along with a question of "how many triangles?" can be found in this image.
Try solving it yourself, if you like, before reading on, where I'll explain for you the correct answer, and show you a fun and beautiful math pattern that's in there, too.
As can be expected, I saw a large number of attempts at answering this, including some fairly sophisticated erroneous ones.
Image credit: source unknown, retrieved from Irena Haj.
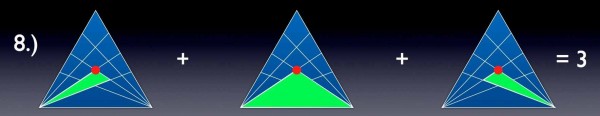
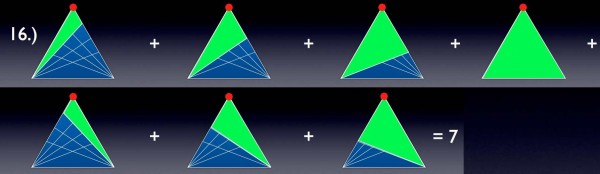
It makes sense to try and construct triangles from each of the points where lines intersect, but you have to be careful not to double-or-triple count triangles. The number here is too high, as the answer isn't seventy.
Image credit: Patryk Solarczyk.
This one was particularly bothersome, because -- spoiler alert -- 64 is the right answer, but this diagram is totally wrong, missing some triangles that are actually there, and counting a number of triangles twice. (For example, look at the fifth row, at the red triangle in the first column, and how that's the same as the green triangle in the sixth row, second column.)
When someone gets the right answer for the wrong reason, it's particularly aggravating, because it takes multiple mistakes to make that happen. So I'd like to show you a foolproof method for showing you all the unique triangles in this diagram, and when we're finished, we'll see a pattern and get a formula to learn something fun and beautiful.
All of the points of intersecting lines within our triangle.
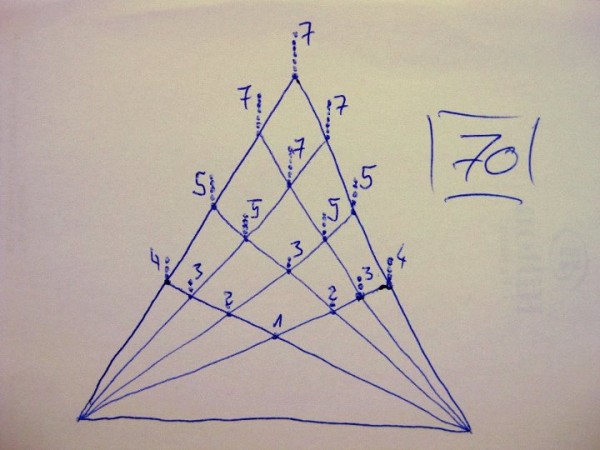
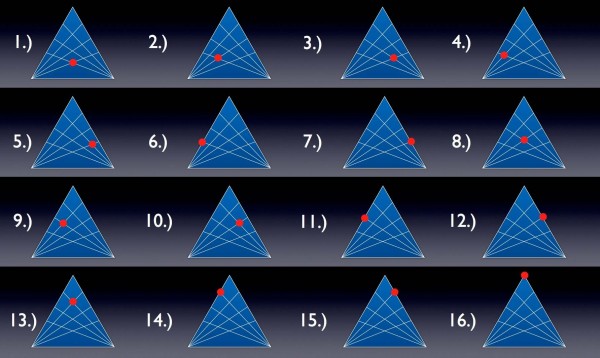
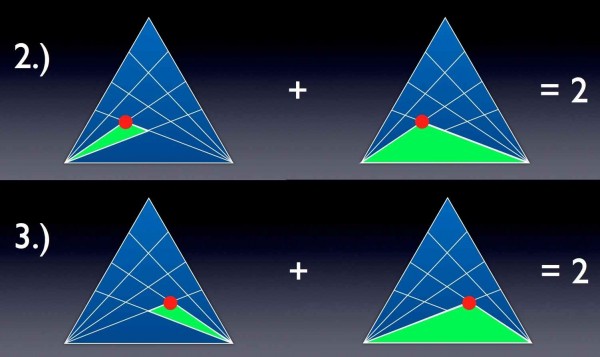
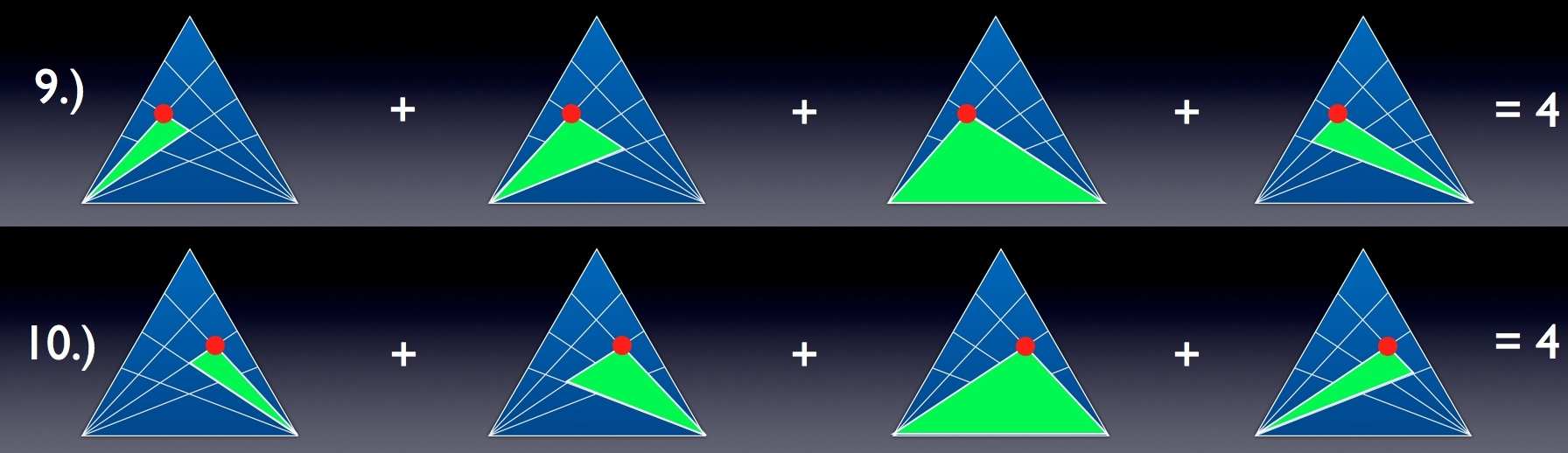
We're going to start at the bottom of the triangle, with the two base vertices. As we move up the diagram, we'll progressively run into points where two lines intersect, labeled above in the order we'll run into them.
Each time we do, we'll count all the new unique triangles by using the new, intersecting point and one (or both) of the two base vertices at the bottom of the triangle. In order to avoid double-counting, we'll only create triangles using points below our current point, ensuring that we'll never count the same triangle twice. You'll also notice that some points -- labeled 2 and 3, 4 and 5, 6 and 7, 9 and 10, 11 and 12, and 14 and 15 -- are mirror-reflections of one another, so those sets better give us the same numbers of triangles.
Let's go through these points, from 1 to 16, and see what we get.
Point #1 as a necessary vertex in each triangle.
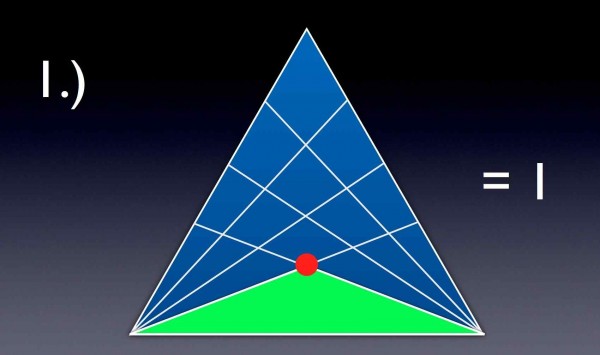
For the first point we come to, there's only one possible triangle using the points below it: there are three points in a triangle and this triangle uses all of them.
Easy enough, so it's on to the next one(s) up.
Points #2 and #3 as a necessary vertex in each triangle.
As you can see, each of those new points can make two new triangles, one using both base vertices and one using our intersecting point #1, which is now an option in making a triangle. This pattern will continue as we continue to move upwards, as all lower points now become fair game.
So let's move up to points 4 and 5.
Points #4 and #5 as a necessary vertex in each triangle.
There are three new triangles we can construct for each of those, as you can see. This is pretty straightforward, as are points 6 and 7, below.
Points #6 and #7 as a necessary vertex in each triangle.
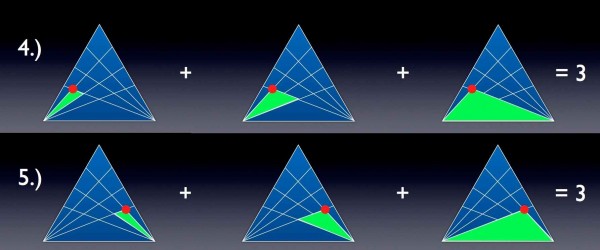
Four new triangles apiece, using all of the allowable, lower points as possible vertices. So far, so good: no double-counting, and no missed triangles. And moving up one more, to intersecting point #8, finally gets a little interesting.
Point #8 as a necessary vertex in each triangle.
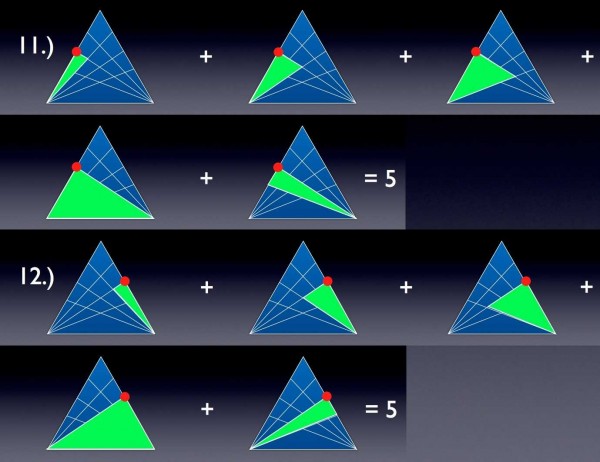
Why is this -- point #8 -- interesting as compared to the others? Because, for the first time, we can build successful, new, unique triangles that connect to either one of the base vertices, something that we'll have to keep in mind for all of our subsequent points.
Let's move on up, and hit points 9 and 10.
Points #9 and #10 as a necessary vertex in each triangle.
Points 9 and 10 give us four new, unique triangles each, connecting to either (or both) base vertex (or vertices), as appropriate.
Points #11 and #12 as a necessary vertex in each triangle.
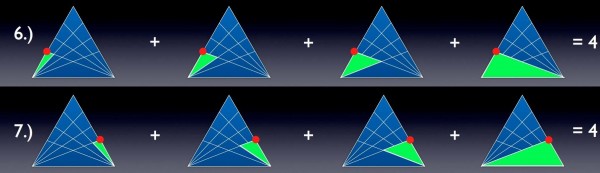
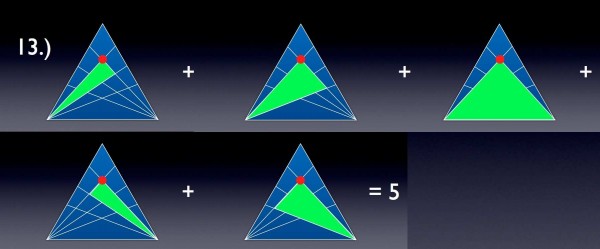
And for points 11 and 12, we get five each. Feel free to check: all of these triangles, so far, are unique, and encapsulate all of them. We've only got four intersecting points left, so let's take them all down!
Point #13 as a necessary vertex in each triangle.
Five more for intersecting point #13...
Points #14 and #15 as a necessary vertex in each triangle.
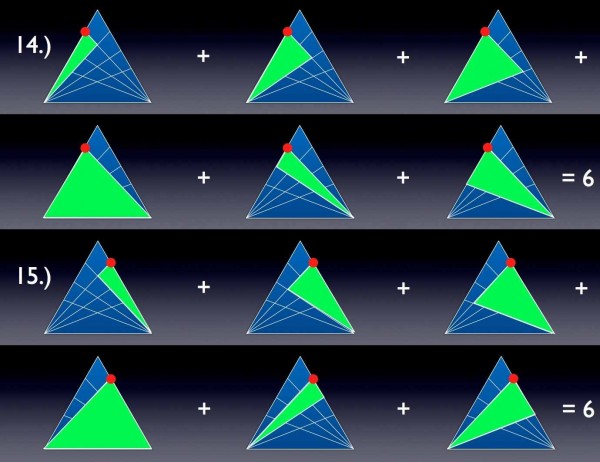
Six each for points #14 and 15, and for the final, uppermost point...
Point #16 as a necessary vertex in each triangle.
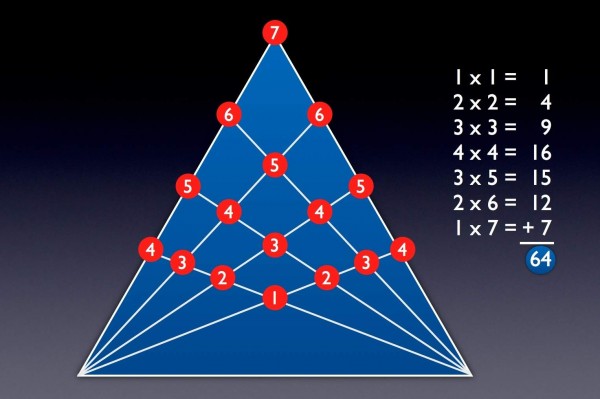
Seven! All told, we can add these up, and get 1 + 2 + 2 + 3 + 3 + 4 + 4 + 3 + 4 + 4 + 5 + 5 + 5 + 6 + 6 + 7 = 64, and so there are, in fact, 64 unique triangles here.
Now, 64 is an interesting number: it's a perfect square (82 = 64), it's a perfect cube (43 = 64), and you might wonder if it's related to the number of extra lines coming out of those two base vertices. Well, it is, but the pattern is really fantastic. Let's show you what we get if we count the number of new triangles we were able to create -- using each new point as a necessary vertex -- as we moved up the triangle.
Number of triangles created at each new vertex, going upwards.
Now, that's a beautiful pattern, and it happens to be very closely related to the number of lines -- in this case, 4 -- coming out of each base vertex of the triangle.
If we only had one, we'd only have the lowest line from each vertex, meaning we'd only get 1 triangle.
If we only had two, we'd have the two lowest lines from each vertex, getting a total of 8 triangles: 1 x 1 + 2 x 2 + 3 x 1 = 8.
If we only had three, we'd get the three lowest lines from each vertex, for a total of 27 triangles: 1 x 1 + 2 x 2 + 3 x 3 + 4 x 2 + 5 x 1 = 27.
And as you can see, for four, we get 64: 1 x 1 + 2 x 2 + 3 x 3 + 4 x 4 + 5 x 3 + 6 x 2 + 7 x 1 = 64.
And, as you may have noticed, 13 = 1, 23 = 8, 33 = 27, and 43 = 64, so that's how the pattern goes! So go ahead and draw a triangle with an arbitrary number of lines coming from each vertex; you'll not only now know the pattern, including how many triangles you can generate as each vertex as you move upwards, but you now know an awesome way to generate the perfect cubes of numbers! What a fun and beautiful little bit of math, and I hope it helps bring you not only a great weekend, but peace of mind, and closure to this epic triangle riddle!
- Log in to post comments
More like this
Ethan, wow, that's really ... wonderfully studious, fun, and enlightening. I'm glad to have inspired you and noted appropriate caveats.
http://tyrannogenius.blogspot.com
I don't see a problem with double-counting triangles in the solution that you think is wrong. Rather, it appears that you are viewing the triangles wrong and are assuming that different colored triangles "overlap" each other, when in fact each triangle is defined completely by a single color. So the red triangle in row five is is a small sub-area of the green triangle in row six. After all, the green triangle is isosceles, whereas the red triangle is clearly not.
Also, the diagram does not show symmetric triangles, but instead multiplies the count by two when appropriate.
The formula is also (using a different counting method):
(n * sum(1...n)) + (n * (sum(1...(n-1))) = n**3
The formula would be
2 *n*sum(1..n) with double counting.
First count all the triangles that have the lower left point as a vertex. For each line that intersects with the lower right point there are 1 + 2 + ... + n that use part of that line to form a triangle. There are n such lines so the number of triangles that have the lower left point as a vertex is n * (1 + 2 + ... + n). From symmetry, there are an equal number of triangles that have the lower right point as a vertex. There has been some double counting but a little inspection will show this can be accounted for by reducing the (1 + 2 + ... + n) term to (1 + 2 + ... + (n-1)) for one the sums.
Greg- That's also the way I saw it at first, however, our interpretation misses triangles and leads to double counting anyway. By that interpretation, the red triangle in row five column one is the same as the green triangle in row one column two.
How about this: Divide the lines into three sets
1) the horizontal line
2) The four lines other than the horizontal that end in the left vertex
3) The four lines other than the horizontal that end in the right vertex
Any combination of lines from a) sets 1,2 and 3 or b) sets 2 and 3 will lead to a single triangle, and all triangles in the figure can be represented by either one of these combinations. (Why? Any three lines will form a triangle unless they are parallel, or all pass through the same point., and all triangles need three lines)
Therefore, the number of triangles = a) 1*4*4 + b) 2*4C2
Since 4C2=24, that gives us 16+48 = 64.
Typo above: the number of triangles = a) 1*4*4 + b) 2*4C2*4
Since 4C2*4=24, that gives us 16+48 = 64.
(Sorry for the multiple posts)
Extending this to the case of n that Ethan mentions is also easy. If sets 2 and 3 have n lines each, the answer will be n^2 + 2*nC2*n = n^2 + 2*(n*(n-1)/2)*n= n^3
A simpler explanation of the formula in my previous comment:
All triangles have either the lower left point or the lower right point as a vertex with some having both points as vertices. So the total number of triangles is
The number of triangles having the lower left point as a vertex
plus
the number of triangles having the lower right point as a vertex
minus
the number of triangles have both points as vertices.
These are n*sum(1..n), n*sum(1..n), and n*n, respectively.
This is equal to n*n*(n+1)/2 + n*n*(n+1)/2 - n*n = n*n*n.
Testing. I can comment here but not on your Empty Space post. If anyone else can post on Ethan's post (Empty Space has more energy than everything in the Universe, combined) please post a test working post there.
In explaining why that first set of diagrams was wrong, Ethan, I think you made a simple typo: "(For example, look at the fifth row, at the red triangle in the first column, and how that’s the same as the green triangle in the sixth row, second column.)"
That should read: "same as the green triangle in the FIRST row, second column.)"
The two triangles to the left of it are also duplicates, of course.
And that same error gets repeated in the next two triangles in that fifth row.
Damn, I just got it. Except in my world, the answer is NOT 64. Go back to that first diagram that gives us 64. We can agree that the first four rows are all valid triangles, with no duplicates -- right? Total so far: 48.
I finally figured out what the guy/gal was trying to do in row 5. The number "4" does not indicate the individual colored triangles. It's the number you get by progressively adding the smaller colored triangles to the green block, which hasn't yet figured in our calculations. You get 4 more more triangles each time the block's boundary moves right.
The mistake the guy/gal makes is not starting that row with the isosceles triangle that starts row 6. That would give us four more triangles. So you get 4+4+4+4, or 16 new triangles. Then add the mirror images, for another 16. That's 32, except subtract one because the filled-in triangle only counts once.
I get 48+31. That's 79. I haven't looked at Ethan's mathematical formula to see where it goes wrong, but I'm pretty sure it does.
Here is a video that explains it:
http://youtu.be/FxByF38grkQ
You may want to pause the video at certain points.
The video doesn't explain it. I can still visually count 71 triangles, none of them duplicates.
Make that 79.
Heh, it's amazing people are still arguing over it. But I think once you get the pattern: work out all possible combinations of perimeters anchored from each bottom corner, times four because of the other lines cutting them off, times two from the other side - then remove the nine duplicates with top points, that are inside the main space (that for example are two sectors from one corner but only one sector from the other, etc.) well that makes 64. But if you can show otherwise, well knock yourself out.
BTW for that example and more, see http://www.facebook.com/enhancegeneralknowledge
Now that I think about it, there are three more center-line "green block" isosceles triangles that cannot be included in the mirror-images calculation. So make my total 76. Anyway, still more than 64.
Hey Neil: I have no problem with being shown to be wrong. All I ask is to be shown exactly where i went wrong. I believe I can identify 48 unique triangles in the first four rows of that initial diagram. I have explained how I see 28 unique triangles in the area that was was not examined up to that point. The triangles identified at either stage do not overlap, so have I miscounted the 48 or the 28? Where am I seeing triangles that don't exist?
Chuckinmontreal,
> "Where am I seeing triangles that don’t exist?"
Draw those extra 12 triangles that you have out on a sheet of paper, and upload the image so we can have a look.
OK, I'm tired and my eyes are getting blurry, but I can now see where several of those last 28 triangles are duplicates, even before counting mirror images. It shouldn't be too hard to whittle that number down to 16, at which point Ethan will be vindicated. I'm glad I didn't use my real name to comment, although I did suggest to a mathematician friend that he visit the site and tell me if I was onto something. Maybe he'll forget to check his e-mail this morning. Move along, folks. Nothing to see here.
Another way to count the triangles is to consider all the triangles made with just two straight lines (and enclosing the space between them, and having no other lines trapped in the middle), then those that trap one line in the middle, then those that trap 2, and finally the single whole triangle that traps three. You can see examples of these in Ethan's final section 16 - the first four triangles progressively show one of each type mentioned above. If you count these (and avoid over counting) you get: no trap - 31, one trap - 21, two trap - 11, three trap - 1 = 64. I like the pattern here as well.
The universe doesn't make sense. Nothing that's built on QM can make sense.... some parts of the universe make sense, that's all.
But then, something which partly makes sense and partly doesn't is neither particularly remarkable not particularly scientific. A shaman can probably predict the weather about as reliably as a meteorologist, but that doesn't make the shaman a scientist, at least in the sense in which William Whewell used the word. Similarly, a Japanese swordsmith uses poetry rather than pyrometers to get the quality of his steel right, with very repeatable results - but is that science?
We're built to notice and use the bits that make sense. Some of us are built to notice and wonder about the bits that don't, like QM. I'd say that the latter are the scientists.
Once you've counted the 48 triangles radiating from one vertex or the other, the visual solution was really much simpler than I made it. All remaining triangles must include one of the four on row 6. The first has three triangular slivers on the left that could be added to it, and three on the right, for a total of seven triangles. The second has four slivers, for five. The third has two, for three. And the filled-in triangle gives us one. That's 7+5+3+1 = 16. Plus 48 = 64.
When will I learn that Ethan Siegel knows all, sees all, and makes only the occasional typo?
Hi Ethan, what software did you use to draw all those triangles?
MadScientist,
Because it looks artistic, I think that he might have done it with Adobe's Illustrator. It would be pretty straight forward; draw a triangle, draw a transverse vector line out of one corner, rotate/repeat/copy it, and end it all off by using the 'Divide' tool in the 'Pathfinder' pallet. Next you can start to color every area as you like.
MadScientist,
I used Keynote, the apple version of Powerpoint. I made the first big triangle using a triangle and some lines, and then screen-captured it and inserted it as a background image for the subsequent, overlaid triangles, red circles, and occasional text.
Nothing fancy.
Thanks Ethan, I'll have to keep that in mind when I make simple diagrams. I have a habit of using a small set of tools and although they're great for complex diagrams or large data sets, they tend to be very complex to drive if I only want something simple.
Thank you for a very interesting puzzle for starting both my algebra and chemistry classes with in a few short weeks. I love the patterns and the reasoning about scientific thinking. And the comment about a shaman predicting the weather as well as a meteorologist throws in a little of the chaos of real life. Beautiful stuff.
I love how, in your final diagram, starting at the vertex labeled "1" all ascending paths through the edges encounter vertices labeled with natural numbers, "1", "2", "3", "4",..."7"
Math and geometry: love it!
theres actually 74 triangles
Great post and love the explanation. We shared this on our Facebook page and received a ton of participation. One math professor in North Carolina (Luke Walsh) even created an interactive graph to help find the solution: https://www.desmos.com/calculator/5s2upiv6xw
Thanks!
Team Desmos
No,.....there are only 8 triangles......7 triangular "tiles" on the inside and the whole triangle make 8.
The rest are 9 - 4 sided "tiles".
You may want to specify that you can "cross over lines" to make your shapes.
All in all,....asking how many trangles in the image is misleading.
I got 61. Here is how:
Here are different triangles outlined radiating from the lower left corner:
http://www.flickr.com/photos/88720457@N04/sets/72157631781907082
You can see that there are 37 triangles. But... there are also triangles radiating from the lower right-hand corner, so it would stand to reason that we could double 37 to get a total of 74. *However*... if we repeat the process, the triangles that have their peaks in the middle (i.e. numbers 4, 22, 34 and 37) are clearly duplicates, so those 4 should be deleted from the total before doubling. So we would then get 37 - 4 = 33. So 66 plus the 4 we took out makes 70. *However*... if we take a closer look, every time a left-radiating triangle touches the lower right-hand side corner, we know that those triangles will be duplicated once the process is repeated... so we can't count those. In total, there are 13 triangles that touch BOTH the lower left and right-hand corners (which includes the middle triangles)... so 37 - 13 = 24. Double the 24 to get 48, add the 13 we subtracted and you get 61.
I fully admit I could have made a mistake... but I have been staring at this and needed to get it "out" :). Thoughts?
And now with a fresh mind: a friend pointed out that I left out the mirror images of 16, 28, and 36. With those, the calculation would be (24 * 2) + 16 = 64. Yay morning tea!
I counted/calculated 64, using the following method.
First I noted that all triangles must use either the bottom left vertex of the outer triangle, or the bottom right vertex, or both (all internal lines intersect one of those vertices).
Based on that, I first counted all triangles that used the bottom right vertex, then all triangles that used the bottom left vertex but NOT the bottom right vertex.
I began by counting all triangles that use a vertex on the lowest line originating from the lower left vertex (that is, all the triangles below that line). Beginning with the topmost vertex on that line, I counted 4. Moving to the next vertex down the line I counted 3. Continuing in this fashion, I counted 2 for the next vertex, and 1 for the final (bottom-most central) vertex.
The total number of triangles below the lowest line originating at the bottom left vertex = 4 + 3 + 2 + 1 = 10. By observation, the number of triangles can be calculated using ∑(1, n), where n is the number of vertices on the line (in this case 4, not counting the lower left vertex).
I then multiplied that by the number of lines originating at the bottom left vertex to get 40 triangles that use the bottom right vertex.
Next I calculated the triangles that use the bottom left vertex but not the bottom right vertex. The base formula for the numbers of triangles below each line became ∑(1, 3), since I couldn't use the bottom-most vertex. ∑(1, 3) = 6.
Multiplying by the number of lines gives 24 triangles using the bottom left vertex but not the bottom right vertex.
Total triangles in the figure = 40 + 24 = 64.
You've all missed the two extra triangles hiding in the letter "A"s in the title.
Hi,
I used this to calculate the number of triangles,
4 + ((( 5 + 4)*4) *2) = 76;
5 is the combination of the bars on one side and 4 is side triangle. Obviously I have duplicates,
Can someone correct me where I duplicated the numbers or need to subtract ...,
Thanks,
Am I the only one who took the old school route, drew it out, and assigned every point a letter? I find it much simpler, for me, as I'm less likely to make a common math error. I just made a list of every triangle I saw (ABC, ABD, etc). Can't go wrong there! (And no mathematical equation necessary)
There is only one triangle rest are his friends... ROFL.. :)
Actually there are 64 triangles
Actually, they are all made up of little squares, so there are NO triangles in that (bitmap) picture!
Thanks! I always look for interesting math/mind problems for my students to tackle on Fridays. This will be a fun one, and I love all of the debate as welll as examples of wrong answers. Very good for asking questions!
80 triangles
There are 76 triangles, I think.
The answer is 64.
Let me name the vertices of main triangle as A,B,C.
B and C be the vertices of base line.
The no. Of triangles using point B are 4*(4+3+2+1) = 40
Similarly the no. Of triangles ising point C is also 40
So total is 80. But this will include some comman triangles.
Common triangles will be with vertices B and C both = 16(easy count)(count the no. Of points other then B and C).
So the answer is 80 - 16 = 64.
Whats so tricky about it .
If doubt u can mail me shreyasbhatt9181@gmail.com
42.
It's always 42.
Unless the question is "what's the password?".
70 is the correct answer.
I'd like to point out that the picture simply asks for how many triangles there are. So the one rule that we must abide by is the fact that all triangles created need to take up a different section or space. If you don't count the same exact triangle more than once and create as many triangles you can, then you will count 33 on each side without including the "centered" triangles. Then you can add the 4 "centered" triangles for a total of 70.
Why does everyone have to complicate the maths??
from the base line there are 4 lines at each side radiating upwards at different angles. these lines intersect at 16 different points (not including the base line). 4 x 16 = 64 or 4 cubed = 64
Simples!
Start with triangles having as a side some segments belonging to the leftmost "big side". There are 10 such segments, 4 of those have the bottom vertex as an endpoint; for each of those 4 segments you have 4 possible triangles you can form with that segment as a side. For the remaining other 6 segments you have one possible triangle each. Next move to the second big side from the left. You now have still 10 possible segments, 4 of those with the bottom right vertex as an endpoint. For each of those segments you can have 3 triangles you can form having such segments as a side. the remaining 6 segments still give you 6 additional triangles. repeat this reasoning 2 more times. there are no more triangles you can form, since if you wipe out the 4 big sides to the left there are no triangles you can form. Hence the answer is (4+4+4+4+6)+(3+3+3+3+6)+(2+2+2+2+6)+(1+1+1+1+6)=64
Why on your diagram (4th image from the top) the fifth row equals 12, if you take out the double triangle that is in the sixth row, wouldn't it add up to 22?(11 x 2)
Giving you a total of 74.
The answer is 70 - I agree with Dude #48 although i came at it from another method.
We surely agree from the Patryk Solarczyk image and solution that the first four lines of anaysis are correct and make 48 triangles total.
Now if we lable the bottom left corner as X and the bottom right as Y on the final Ethan Siegel image and follow
from X through 1-2-3-4 to Y and from Y through 1-2-3-4 to X
that provides 7 unique triangles as X-1-Y is common.
then progressing
from X through 2-3-4-5 to Y and from Y through 2-3-4-5 to X
produces a further 7 unique triangles
as does X-3-4-5-6 to Y and Y-3-4-5-6 to X provide a further 7 unique triangles
therefore 48 plus 21 plus the largest triangle X-7-Y produces the correct answer of 70.
My post is over-complicated, I like Dude's clear and concise explanation at #48 best.
Mea culpa - I see the duplications in my method.
The number of triangles off each vertex is the best method per Ethan's final image - doh!
I'll get me coat
The correct answer is seventy six 76 only.
god afternoon
sory , for wrong answer . but the true one is 64 sixty four
happy day
Over complication. No need to count triangles! Look, you need only count peeks(points) and center valleys(intersects), then some simple arithmetic. In this particular case: 16(3+1). Allow me to elaborate: [all vertical peaks x (center valleys + uppermost peaks)]. That is it, simple! What is more interesting is the rate of increase we have. We will look at some ordered pairs which are listed by number of triangles contained. We will start with 0 in the assumption that no triangle will afford no contained triangles. In the case of 1, we have only the bordering or the "container" triangle, which will allow only 1 contained triangle. Our second, will have one "X"(center valley/intersect) and it will contain 8 triangles; to include our bordering triangle(we always count the bordering triangle as a "contained" triangle). Our ordered pairs for no triangle to a triangle containing 5 "X"'s or center intersects: (0,1,8,27,64,125,216). We see an increase of (1,7,19,37,61,91). Our rate of increase in ordered pairs looks like so: (6,12,18,24,30). Our rate of increase is 6:1. This allows us to eliminate counting any peaks and valleys and count an infinite number of triangles with an infinite number of "X"'s in our "container triangle".
Thanks, Robert.
I ran through 6 intersects and I'll do 7-10 later. As it turns out, an even easier way to deduce this pattern would be instead of labeling the center intersects, valleys, just focus on the centermost peaks or points. If we were to draw a vertical line from the point of our container triangle down to the base of our container triangle, we would be running into our centermost intersections, or the middle of our "X"'s. Essentially, if we were to fold our pattern in half, we would easily see the centermost triangles points. In this example, we have 4 center triangles. All we need to do is cube that number: (4x4x4). This works all the way to seven so far.
Thanks again, Robert.
this was my attempt ;0, i guess i was wrong lol http://gyazo.com/e3f517c4e9e55b524a1b11917847fad7
After drawing out each individual triangle I saw and making sure not to duplicate any, I counted 72. All the formulas and equations I read here make perfect sense though. Weird.
thnaks!!
You are making it so complecated it is just the number eight nobody cares about the science of diamonds!!!
I have a solution that can scale to more lines.
http://www.jjward.com/images/uploads/D8BCEB8B-55D4-CCEA-20CD-AE38DB440D…
GREAT explanation... I counted 26 when I on my own :P. Honestly though, I'm more surprised that 7.4% of people got it right than 92.6% got it wrong! It was pretty hard. But on Facebook, the land of infinite stupidity, everybody was saying 8... *sigh*
there is error in 5th row, it should be 12x2, and not 4x3
So the answer is 76
I agree with Sunny!
In the green/yellow/red/blue diagram break down, the first three rows are doubled to account for mirror images. On the next line, it only indicates 4x3 (I'd consider this the 4th row, since there are only 3 above), but DOESN'T account for the mirror images.
I'm coming up with a pretty confident 76!
Also, looking at the 4th line in that 'colorful' diagram, they didn't account for three triangles created by the line that creates the right side of the small isosceles. If we call this group the base line triangles, it would be [ ( 4 x 4 ) x 2 ] - 4 .... where the ' x 2 ' accounts for mirror images and the ' - 4 ' accounts for subtracting all four isosceles triangles (or the bottom row) which are the only duplicates when using this system. So, 24 + 8 x 3 + 28 = 76.
Aha! I reconciled the top method with the intersection points method and discovered the error of my ways thru the process of elimination! In the base line triangles, there are less mirrors that haven't already been located as you progress upwards. There are only 16 triangles that use the baseline, including the 3 isosceles and 1 equilateral triangle. Great method for solving a tricky problem!!
I read this article inside and out, and I see that a lot of other triangles were missed. I analyzed the image, adn you can see, that even though the count is broken down, that a lot of other triangles are simply not included. I count 131.
Thank you. This was driving me crazy because I thought I should be able to count it and I kept getting different answers.
How complicated... How about (1+2+3+4)^2 - (1+2+3)^2 = 10^2 - 6^2 = 100 - 36 = 64
In summary, we count all combinations of lines leaving from base vertices and remove the combinations that do not form triangles.
I prefer to build outward from the lowest non-base vertex. With that vertex (n=1), you have one isosceles triangle. For the next step (n=2), you add an enclosing triangle, with vertex #8 (in the blue diagrams) at the top, adding one new triangle, and vertices #2 and #3, on the sides, each of which adds 3 new triangles, for a total of 8. Now draw another enclosing triangle, adding (in order) vertex #13 on top, #4 and #9 on the left, and #5 and #10 on the right. Vertex #13 adds 1, #4 and #5 add 4 each (3 using both base vertices and 1 using #13), and #9 and #10 add 5 each for a new total of 27. Now we see the pattern: n=3 adds the final enclosing triangle (vertex #16), and adds #6/7, #11/12, and #14/15 on the sides, with these pairs adding respectively 5, 6, and 7 triangles for each new vertex. Here are the added vertices for each step:
n=1: 1 = 1
n=2: 1 + 2*3 = 7 (8 total)
n=3: 1 + 2*(4+5) = 19 (27 total)
n=4: 1 + 2*(5+6+7) = 37 (64 total)
n=5: 1 + 2*(6+7+8+9) = 61 (125 total)
and so on.
i got 106
there are 76 triangles in this image, I can prove it
but too lazy to make a large post with all images
sorry but 76 is the correct answer
wrong answer ..forgot count single triangle at left side..which lead to more triangle...
(i) first single go up second go up third go up fourth ....simlilar to right side
The correct answer is 72. I understand how the formula works, but it's wrong. I am pretty disappointed because I used this as a challenge at my party and assumed it was right without checking. So I ended up having to scratch the whole challenge and figure out the correct answer after the party had ended. I had found the same answer of 64 on 3 separate online sites so I figured it was legitimate, but yeah anyone can prove there's more than 64 triangles just by doing it visually.
Go ahead, Dillon.
Pass on the visual proof to Ethan.
My son Tejas, 14 years old has invented formula to calculate number of triangles, which works even if number of lines are different from each vertex. He is math genius and tooh three hours to develop this formula and I strongly feel it is unique as not able to find same on internet. Cube formula doesn't work if number of lines are different if number of lines from each vertex are different. If you are interested please contact me on prashantg103@gmail.com
I have been forwarded with too many puzzles on triangles and being frustrated that my answer was always wrong, I came up with a formula.
Identify
Total number of unique straight lines in the triangle
All intersection points with more than two lines
Total number of lines parallel (or not touching within the triangle)
Steps:
1. Calculate max number of triangles all 'N' lines can produce = Nmax = NC3
2. Calculate sum total of max triangles using number of lines (n) passing through each intersection with more than two lines = ∑nC3 (for intersections with more than 2 lines), where n = number of lines passing through that intersection > 2
For example, if an intersection has 5 lines, the total no. triangles from this intersection point is 5C3 = 10.
3. Calculate total number of non intersecting lines sets - if two lines dont meet then treat it as one set = S
The formula is
Total triangles = NC3 - ∑nC3 - (S*(N-2))
N - total number of lines
n - number of lines at any intersection with more than two lines
S - total number of a set of non intersecting line
Using the formula in the above example
Step 1
N = 9 lines
Nmax = NC3 = 9C3 = 84
Step 2
Total intersections with more than two lines 2
Intersection 1 (bottom left corner) - n1 = 5 lines
Intersection 2 (bottom right corner) = n2 = 5 lines
∑nC3 = n1C3 + n2C3
= 5C3 + 5C3
= 10 + 10
Step 3:
No. of non intersecting line sets = 0
S* (N-2) = 0 * (9-2) = 0
Total number of triangels in the above picture = NC3 - ∑nC3 - (S*(N-2))
= 84 - (10+10) - 0
= 64
To add to my above comment:
1. Maximum number of triangles and any 9 lines can create cannot be greater than 9C3 = 84. Therefore, if counting beyond 84 you are definitely duplicating.
2. If three or more lines go through the same point those lines cannot contribute to a triangle. (which is my second step above)
3. If there are two lines not intersecting with each other, they arent going to form a triangle (atleast within the picture), which is my third step.
Max triangles - triangles that cannot be created by more than 2 intersecting lines - triangles that cannot be created by non-intersecting lines
More generally L * R * (L + R)/2 where L and R are the number of rays from each base vertix excluding the base line. I this case R=L=4.
ok my final count is 75 i believe. 9 from each of the 4 rows on each side (36x2) then the 4 up the middle which makes 78 but because i repeated the bottom triangle once and the whole triangle twice i must subtract 3. some of them overlap but they do not repeat in my method, there are two sets of the three tringle on each side and 3 sets of the 2 triangle on each side
(10x4)x2 - 1 = 79. All triangles must have either the lower left or lower right corner. For each corner there are ten pairs of adjacent sides, and four possible opposite sides. Subtract the one duplicate.
Oops. Forgot duplicates...
It's 10*4 for the lower left corner, and 6*4 for the lower right.
64!