"We shall not cease from exploration, and the end of all our exploring will be to arrive where we started and know the place for the first time." -T.S. Eliot
It's been a remarkably exciting week for science, and you've had a lot to say about our new articles at the main Starts With A Bang blog. After all, it isn't every week that we possibly discover the imprint of gravitational waves on the Cosmic Microwave Background!
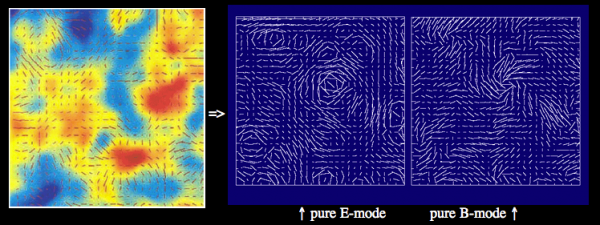Images credit: Seljak & Zaldarriaga (L), Wayne Hu (R), via http://cosmology.berkeley.edu/~yuki/CMBpol/CMBpol.htm.
Let's dive right in -- and respond -- to your comments of the week!
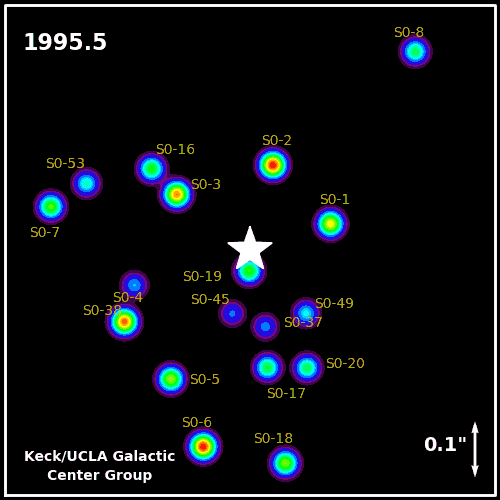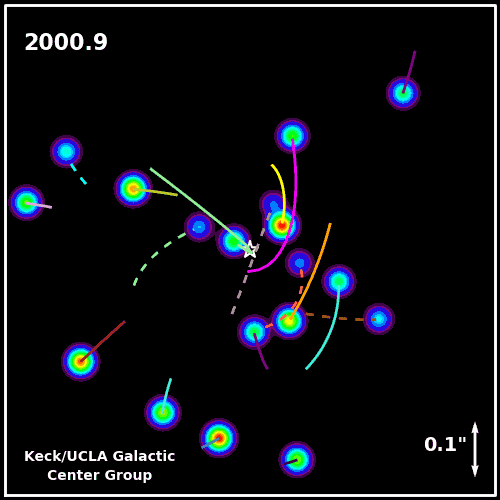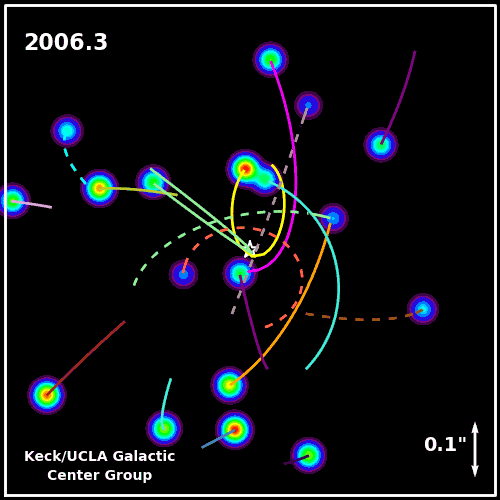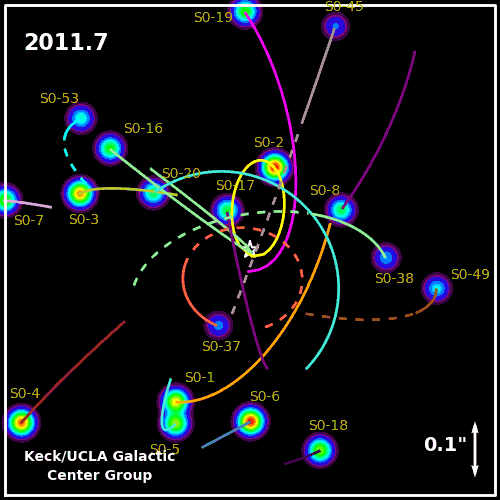
From Michael Kelsey on the topic of Ask Ethan #28: Feeding the Monster: S-2 already made a pericentric pass in 2002, didn’t it? Has the technology improved enough that we will learn substantially more this time than last? Or is it just that we have better focused questions to ask, and can therefore choose particular instrumentation to use?
If you're looking at the supermassive black hole in the center of our galaxy, you won't find any light coming from it. But if you look in the infrared, you can find the light coming from stars that orbit it! Pay close attention to the one labeled "S0-2" in the animation, below. (It's also known as "S2", for "source 2".)
Image credit: KECK / UCLA Galactic Center Group / Andrea Ghez et al.
With an orbital period of just under 16 years around our supermassive black hole, and an eccentric, elliptical orbit to boot, it has, in fact, made one close pass to our galaxy's central mass source, back towards the end of 2002. There's another star, discovered just two years ago -- S0-102 -- that has an even shorter period orbiting the galactic center. The thing is, S2 is incredibly bright (it's a B1-class star), it makes a very close approach to this 4.3 million solar mass object (at just four times the Neptune-Sun distance, or 120 AU), and at the moment of its closest approach, it will be moving at around 5,000 km/s, or nearly 2% the speed of light.
In other words, this is the perfect regime for observing relativistic effects! We did, in fact, learn a lot from the 2002 pass, but you must realize that instruments improve a lot in 16 years! We won't have the James Webb Space Telescope online quite yet, but now that the orbit is precisely determined, we can look for the relativistic redshift during closest approach, something that Keck and the VLT combined are expected to measure to 5-sigma in 2018.
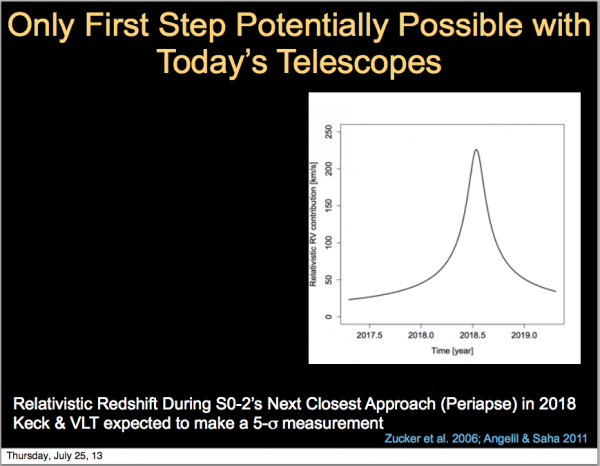Image credit: Andrea Ghez, 2013, slide via http://conference.ipac.caltech.edu/tmtsf2014/system/media_files/binarie….
So, both: we have better instruments (although the JWST and the proposed Thirty-Meter-Telescope could do much better than present technology), but we also know what to look for to very high precision now!
From D.C. Sessions on last week's Comments of the Week:
On average, the Earth takes about 58 days to traverse the equivalent of the Earth-Sun distance, and on average, particles ejected from the Sun take about 3 days to reach the Earth. In other words, the Solar Wind travels more than ten times as fast as the Earth orbiting the Sun.
Am I missing something here? Last time I did any classical mechanics, forces exerted as a result of orthogonal flows are pretty much independent. In other words, no matter how fast the solar wind is moving, the orbital drag is a function of orbital velocity and the density of the matter swept up by the Earth as it goes through the neighborhood.
Otherwise, I don’t see how you’d conserve angular momentum.
There's a funny thing about orbits, relevant to particles ejected by the Sun colliding with our planet. Imagine what would happen if you threw a piece of putty outward, from the Sun, and it collided with the Earth.
Image credit: Daily Mail Reporter, via http://www.dailymail.co.uk/sciencetech/article-1192274/Earth-collide-Ma….
When you think about something colliding with our Earth, you must remember this is an inelastic collision, and that even though its linear momentum might be perpendicular to the direction of our orbital motion, the combined system at the end is either going to have greater kinetic energy-to-mass ratio or a smaller one after the collision.
If we wind up with a greater amount, we'll be more loosely gravitationally bound, and therefore will experience an increase in our semimajor axis, while if it's lessened, we'll be more tightly bound, and will wind up orbiting closer. It's not a flow pushing us out, it's a series of sticky particles colliding with us, giving us an extra, energetic kick and pushing us into higher-energy orbits.
Image credit: ALMA (ESO/NAOJ/NRAO), via http://www.eso.org/public/images/eso1239a/.
That's why we'll spiral outwards as our Sun progresses to (and lives in) its red giant phase!
From Michael Fisher concerning the globular cluster Messier 68: Such globular clusters that are low in elements above lithium… Little chance of Earth-size rocky planets? Would stars in these clusters lack outer regions [sort of beyond 1.5 AU] containing water ice, comets, asteroids & other orbiting objects of interest?
Globular clusters are typically ancient and low in heavy element concentrations; with less than 1% the amount of heavy elements our Solar System has, the stars in this one are among the most metal-poor ones in the local Universe!
Image credit: user Antilhue from Chile, via Astrosurf, at http://www.astrosurf.com/antilhue/m68.htm.
But how little is too little to form rocky planets, or to have a frost line in a star system? How dense and chaotic an environment is too dense or chaotic to have a Kuiper Belt or Oort cloud around a system? We don't have sufficient observational evidence to know where those lines are, but 1% of what we have here is still quite significant! Consider that our Moon is just 1.2% the mass of our Earth; if we had a Moon-sized object orbiting a star in the inner Solar System, we'd certainly consider it a planet, wouldn't we? Consider that Jupiter is 318 times the mass of Earth; wouldn't it be conceivable to get an Earth-mass planet in that system?
Image credit: NASA / Dana Berry.
My hunch is that in a loose globular cluster like this, we would have both Earth-sized planets and -- for many of the stars inside -- frost lines and abundant comets. (Although, I also wouldn't be surprised if overlapping Oort clouds didn't lead to a spectacular cometary show early on in the life of a globular cluster, and that they'd be much rarer today...)
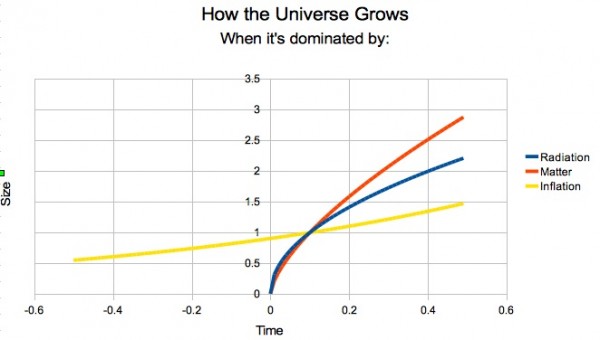
From Algernon concerning All About Cosmic Inflation: do we actually have quantitative estimates about the inflation epoch – i.e. size of the universe when it started/ended, time when it started/ended, energy scales involved, etc.? E.g. where does that 10^-32 seconds come from…?
So if you read and understood what I was writing about, you probably are even more confused about (incorrect) timelines like the one the NSF shows about the history of the Universe.
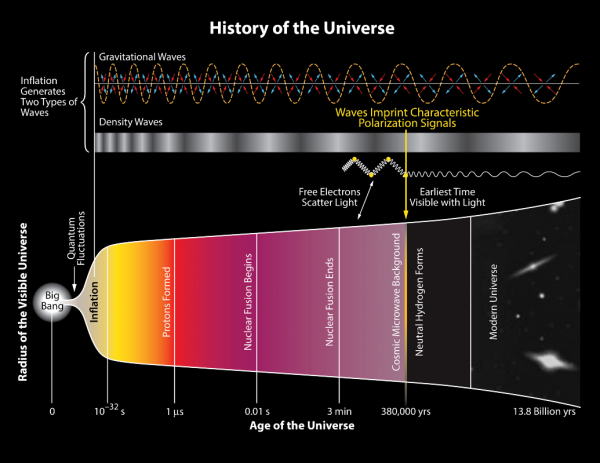mage credit: National Science Foundation (NASA, JPL, Keck Foundation, Moore Foundation, related) — Funded BICEP2 Program.Note that this diagram messes up the Big Bang coming after inflation on their timeline of events in the Universe.
Why do I take issue with this? If you naively extrapolated backwards to an infinitely hot, dense state, you would have had a singularity at the time "t=0," assuming that today the age of the Universe is our "t=13.8 billion years." But if cosmic inflation happened and set up the Big Bang, this is no longer true. In particular, there's no longer a singularity, and there's no longer any special meaning to "t=0", other than the fact that the part of the inflationary epoch whose gravitational waves and density fluctuations are stretched across our Universe today came from those first 10^-30something seconds.
Image generated by me, of the scale of the Universe (y-axis) vs. time (arbitrary units).
Where did inflation come from? How long did the inflationary epoch last? Was there a singularity before inflation got its start, or was inflation eternal to the past?
We don't know, and by the very nature of inflation -- by the fact that it wipes out whatever information was present in the Universe before the final 10^-30something seconds of inflation and its transition to the hot Big Bang -- we may never be able to answer those questions, because that information doesn't exist in our Universe. But that's why I think it's so important to be correct and precise about what we do know.
And our final question...
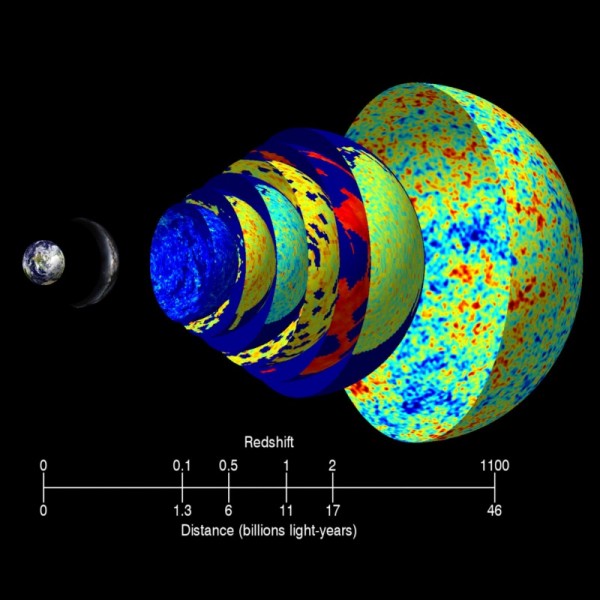
From Anne Blankert as respects The Cosmic Speed Limit: Besides the concept of fixed maximum speed, another concept of relativity is that speed can only be measured (exist) relative to something else. Is the cosmic background radiation some sort of fixed reference frame so that it will fry us when we speed up relative to that?
The cosmic background radiation is the radiation that the Universe emits -- or rather, that free-streams from a surface of last-scattering -- when electrons and atomic nuclei finish combining to form neutral atoms. It comes to us in all directions, with a uniform temperature of around 2.725 Kelvin, that continues to drop as time goes on.
Image credits: Earth: NASA/BlueEarth; Milky Way: ESO/S. Brunier; CMB: NASA/WMAP.
But this doesn't pose a problem for relativity at all; on the contrary, in the context of general relativity, we can use the temperature imperfections in the cosmic microwave background to figure out what our own peculiar velocity is with respect to the Hubble flow. We find -- just as we expect -- that the light from the CMB is redshifted slightly in one direction, and blueshifted slightly in the opposite one.
![Image credit: Delabrouille, J. et al. arXiv:1207.3675 [astro-ph.CO].](/files/startswithabang/files/2014/03/figures_dipole_map.png)
Image credit: Delabrouille, J. et al. arXiv:1207.3675 [astro-ph.CO].
All told, we have a cosmic motion of around 670 km/s relative to this rest frame. But if we wanted to fry ourselves, we'd need to be moving awfully fast; even moving through the Universe at 99.99999% the speed of light would only turn the CMB into the energy equivalent of sunlight, so we've got a long way to go before we do anything that fries us!
Hope you enjoyed this edition of Comments of the Week, and looking forward to some good ones for next week!
- Log in to post comments
Thanks, Ethan! The relativistic redshift measurement is cool, and its clearly something we couldn't have done last time (since we didn't have the orbit of S-2 back then).
You quote a value of 670 km/s for our peculiar velocity relative to the CMB. Wikipedia (https://en.wikipedia.org/wiki/Cosmic_microwave_background#CMBR_dipole_a…) says 369+/-0.9 km/s (that value comes from Planck 2013 XXVII). Coincidentally, the color scale on the figure runs from -3354 to +3354 uK; I don't suppose the conversion from uK to km/s is roughly 0.1, is it?
Michael,
The 670 km/s includes the speed of the Sun around the center of the galaxy and our galaxy's motion in the local group. I believe the 370 km/s value quoted is with all that subtracted out, and is just the peculiar velocity of the local group with respect to the CMB. So I think that's interesting!
The conversion is because 1 km/s is about 1/300,000th the speed of light, and the CMB is about 3 K (to 10% accuracy) so 1/300,000th of that is about 1 microKelvin. So, for the CMB, that's pretty close!
A couple of questions that I've wondered.
1. Given the acceleration of the expansion of the universe, at some point in the future, won't the rate of expansion equal that seen during the inflationary period. Would it not be possible for the universe to be cyclic in this form? Or there a problem related to the required amount of energy?
2. Isn't gravity a measure of the gradient of space-time, kind of like a measure of the "rate of change" of some intrinsic field that represents mass? Why is it a fundamental force? I assume I'm missing something really obvious due to a lack of education in the area.
@Robert
1. No, because the rate of expansion is tied to the energy density of dark energy itself. This density is constant at present, so the expansion rate is constant. Expansion rate is not speed. Distant galaxies will move faster and faster from us.. this is that acceleration, but rate at which that happens will be constant.
2. Yes, you are correct. And if you want to be really strict, it's not a fundamental force as electric/magentic/nuclear forces. But on the other hand, every QM theory says that we should be able to quantize it, or ascribe a particle to it (graviton). So.. at the moment.. it's an undefined region weather gravity is force or just a measure of gradient. Certainly in pure relativity it's not a force but a measure of properties of spacetime.
@Ethan #2: I guess I'm even more confused now.
Planck XXVII (2013) says their measured value of 369+/-0.9 km/s (which also shows they're not great with sig figs :-) ), is specificially the motion of the Solar System barycenter with respect to the CMB rest frame.
Wikipedia says the derived value for the Milky Way in the CMB frame is 552 km/s (note that value is quoted from COBE; I don't know whether there's a better recent value), and that the net motion of the Milky Way toward the Great Attractor is 670 km/s.
The combination of those last two values tells me that the Great Attractor itself must have some non-zero peculiar motion w.r.t. the CMB frame.
The one thing all of this does say, and *should* say to the other readers, is that special relativity is alive and well. We really can't point to anything and say it's "absolutely at rest," not even the CMB! All measurable motion is relative, and whatever we call "at rest" is merely for our convenience in a particular observation.
Michael,
My apologies; I checked and I'm backwards! 370 km/s is our measured dipole anisotropy with respect to the CMB, 670 km/s is the overall peculiar velocity of the Milky Way with respect to whatever the great attractor is. So my explanation is backwards, as your cites correctly point out!
Yes, of course, special relativity is alive and well, and having a cosmologically "preferred reference frame" at the hands of the CMB does nothing to negate it at all.
One interesting question which is often overlooked (because it's unsolvable) is to ask how much of the dipole anisotropy is due to our peculiar motion, and how much is primordial, much like the other anisotropies in the CMB. We may never know unless a unique, clever primordial disambiguation method is devised. In the meantime, we simply subtract the whole thing out. But as you (perhaps) rightly point out, the uncertainty there is greater than the equivalent of 0.9 km/s!
Thanks again, Ethan! Hmmm, "unsolvable." Maybe, maybe not. It certainly seems impossible to separate out components of a simple dipole. But then, I think some would have argued that measuring the B-mode polarization would be "impossible" (too small to detect above the noise). And observing the event horizon of an astrophysical black hole is surely impossible (until we build the EHT).
I don't see how you can be wrong, but I don't want to completely write off the inventiveness of good experimentalists :-)
Thanks for answering my question, Ethan.
That yellow line on your graph suggests quite a big paradigm shift it seems, one that wasn't specifically discussed by other blogs commenting on the announcement.
Assuming BICEP2 is confirmed, do you think it's possible to achieve, during the next years/decades, experimental confirmation of a specific inflation model, among the many available, that actually helps us understand how to properly draw that yellow line, i.e. extrapolating back to times that have left no trace in our Universe? Finding the actual potential of the inflation field, is that doable? Would it suffice?
@algernon #8: One of the interesting consequences of the BICEP2 result (if it is correct), is the a very wide range of different inflationary models are ruled out, primarily because they either predict no stochastic gravitational radiation, or predict it at levels below the R(t/s) ~ 0.2 level BICEP2 is claiming.
You say, "left no trace in our Universe." By definition, anything which "leaves no trace" is unobservable, and therefore untestable. Inflation generically *does* leave traces: the simplest being the 10-5 uniformity in the CMB across the observable universe (impossible unless those points were causally connected in the past); the B-mode CMB polarization amplitudes being more complicated.
Any model which makes predictions which do leave "trace[s] in our Universe" is testable, and may either be supported or ruled out by future measurements. If we're really lucky, a concordance of such measurements will all support a single model, which we will (eventually) consider confirmed.
If we aren't so lucky, we'll be left with the same 10^500 possible string theory parameter sets, anthropic arguments for the multiverse, and all the rest of my theorist colleagues' paper-generating jargon. (Bitter? Nah, I'm not bitter :-) ).
What this will do is to finally allow some real numbers for inflation theories. This is huge. This is like what CMB was back when it was first imaged.
Also it will probably allow for much more specific info on inflaton as a particle.
So yes, my bet is that in 5-6 years time, we'll have a much more precise picture of what happened at those times. And am also betting there will be some pretty awesome, never even thought, things.
Is it possible that the BICEP2 results (assuming they are confirmed) will give more insight into the precise nature of dark energy? I may be misunderstanding this, but is it not true that dark energy is responsible for the observed accelerating universal expansion? If so, is it way off base to think that the current expansion might be related to inflation? It seems reasonable (unless I am way off base here), then that an improved model of inflation might well tell us something about dark energy as well.
@ Sean T
Yes, of course. Dark Energy is that non-zero leftover potential from inflation.
p.s.
... or rule it out completely, of course. Highly unlikely IMO, but it's a possibility.
Ethan, if 99.99999% c makes the CMB as powerful as daylight, how many more 9s before it really does destroy any matter?
Thinking that would be the cosmic speed limit for starships...
@Mark M #14: See also Ethan's post http://scienceblogs.com/startswithabang/2014/03/20/throwback-thursday-t….
The answer to your question is the "GZK cutoff" (search that in Wikipedia). It is the energy (a few x 10^19 eV, or about 8 joules!) at which cosmic rays see the CMB as gammas with enough energy for pion photoproduction (i.e., the particle interaction p + gamma -> p + pi0, or p + gamma -> n + pi+).
If, somewhere in the Universe, a proton cosmic ray is produced above the GZK cutoff, it'll hit a CMB photon, producing a pion and a proton with energy below the cutoff.
So, your speed limit for starships would correspond roughly to the speed of GZK protons. The speed is given by sqrt(1 - m^2/E^2) (since E = gamma*m); the proton mass is about 1 GeV, so you've got sqrt(1 - 1e-20), which means nineteen 9's.
Of course, the pion production threshold is about 200 MeV (gamma energy). You're going to start taking serious damage when the CMB looks like X-rays, i.e., a tens of keV. So the energy limit is probably about three or four orders of magnitude lower, let's say fourteen 9's :-/
Thanks Michael, appreciated.
Wondering what shielding would work best for ultra-relativistic space travel - a massive tank of water? Or maybe just thick ablative armour, X inches wears off per light year, etc.
(I guess particles and dust could be vastly more damaging than the CMB anyway)