"According to the special theory of relativity nothing can travel faster than light, so that if light cannot escape, nothing else can either. The result would be a black hole: a region of space-time from which it is not possible to escape to infinity." -Stephen Hawking
You may have encountered objects that are the same size as one another, but have very different masses.
This is because they're made out of different elements. The higher you go in the periodic table, the larger and more massive your individual atoms are, and so typically -- neglecting differences in the sizes of the electron shells -- the heavier each individual atom is, the more dense a material is.
But we can do better, in terms of density, than just increasing the mass of our atomic nucleus.
Gravity is -- on the largest scales -- the most powerful and irresistible of all the forces. If it weren't for the intense conversion of matter-into-energy going on in the core of the Sun, our star -- a full 300,000 times as massive as Earth -- would contract down to be no bigger than our own planet. No bigger in terms of size, that is, but it would be thousands of times more dense than even the densest element on our planet.
That's because gravity can compress the very atoms themselves, and it would only be the quantum pressure of the Pauli exclusion principle that kept this hypothetical white dwarf from collapsing farther. If there were enough mass to force the electrons into the nuclei themselves, we could fuse all the protons and electrons into neutrons, creating an even denser form of matter known as a neutron star.
Whereas a white dwarf might be an object the mass of our Sun compressed into the size of the Earth, a neutron star is that same solar mass compressed into a size smaller than New York City! It might be surprising, but an object as massive and dense as a neutron star would be extraordinarily difficult to leave. Here on the surface of Earth, you need to reach a speed of about 25,000 miles-per-hour (or about 11.2 km/sec) to escape from Earth's gravitational pull, but at the surface of a neutron star you'd need to move at around 200,000 km/sec, or more than half the speed of light!
In fact, if you just piled more and more mass atop that neutron star, the individual neutrons would eventually collapse, and not even light would be able to escape. As Hawking (and others before him, going all the way back to John Michell in the 18th Century) have noted, this would create a black hole in space, where matter (and other forms of energy) could fall in, but nothing -- no matter, no light, no nothing -- could get out.
But if nothing can escape from black holes, not even light, then how do we detect them?
The simple answer is: from their gravity.
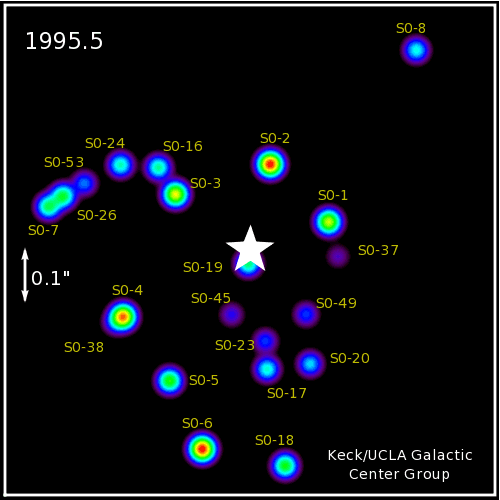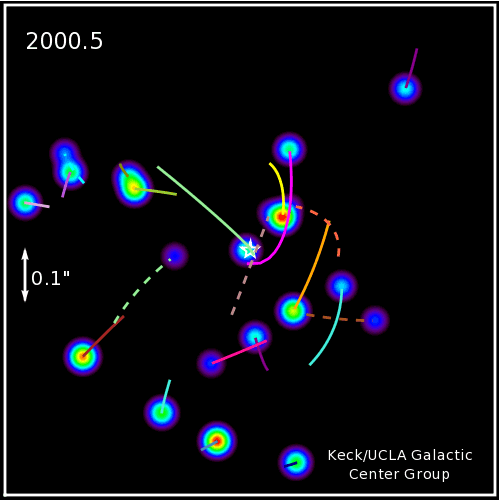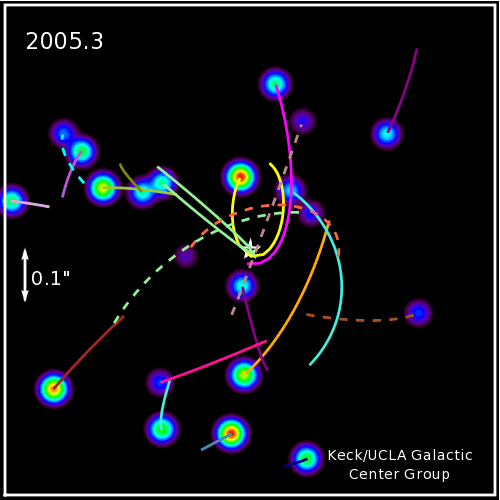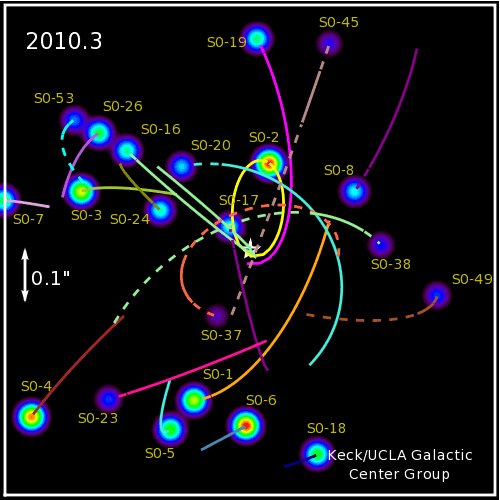
By observing how individual stars orbit a point mass that gives off no light, we can infer that -- at the center of our galaxy -- there's a point mass many millions of times the mass of our star. It emits no light and has no emission signatures of any type.
But this is not the only black hole we know of. We know about the central black holes of many hundreds of galaxies, all of which are too far away to measure individual stars moving in orbit around them. So how do we know they're there?
Because black holes exert intense gravitational forces, they can tear matter apart that passes too close. This includes gas clouds, asteroids, planets and even entire stars, as shown above!
Black holes, like neutron stars, white dwarfs and normal stars, also have strong magnetic fields that get even stronger the closer you get to the event horizon, or the point from which light cannot escape. As matter -- which is made of charged particles, remember -- moves through this magnetic field, it accelerates, emitting radiation of progressively higher and higher energies the stronger the field gets.
So what we can do is look for X-ray emissions from the centers of galaxies, and the accompanying presence of bipolar jets, like those coming from Centaurus A.
 Image credit: ESO / WFI (visible); MPIfR / ESO / APEX / A. Weiss et al. (microwave); NASA / CXC / CfA / R.Kraft et al. (X-ray).
Image credit: ESO / WFI (visible); MPIfR / ESO / APEX / A. Weiss et al. (microwave); NASA / CXC / CfA / R.Kraft et al. (X-ray).
These jets are evidence for a supermassive black hole that's actually active, or presently feasting on some type of unsuspecting matter from within its own galaxy!
The giant elliptical galaxy above, Messier 60, has a multi-billion mass black hole at its center, which we can tell thanks to its X-ray emissions. How can we tell its mass? Because there's a relationship between the X-rays emitted and the mass of the black hole accelerating the matter!
While it's true that not every black hole is active, every black hole that exists in the vicinity of other matter (which is practically all of them) is thought to have an accretion disk. And if we could get close enough to that disk to see it, we'd find that as the matter in it accelerates, it emits progressively more-and-more energetic light!
In other words, the outermost portions of the accretion disk would be invisible, but as you moved inwards, even though the black hole itself wasn't emitting any light, you'd see the accretion disk begin to glow a dim red at some finite radius, and intensify to orange, yellow, white, and eventually blue and violet as you moved inwards towards the event horizon!
If you could see into the ultraviolet or X-ray, those would intensify very close to the event horizon itself, and so even a black hole that wasn't in the process of devouring anything would still be visible thanks to this disk!
But what if you had a black hole that wasn't eating anything, with no accretion disk, and in total isolation from anything and everything else in the Universe? Could you ever "see" it then?
The answer, believe it or not, is yes. You'd just need the right kind of eyes.
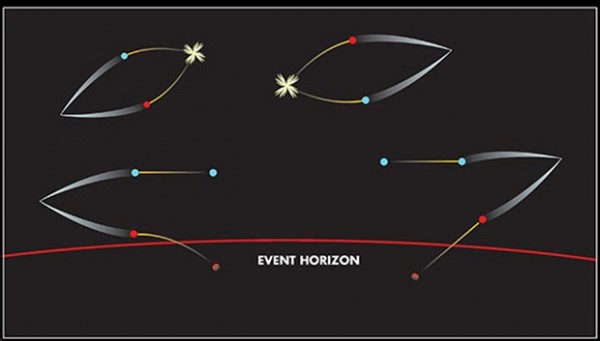
The quantum vacuum is constantly creating particle-antiparticle pairs, that are winking in-and-out of existence. This includes pairs of photons, which we normally gloss over. But when this happens at the edge of a black hole, sometimes one of those virtual particles gets sucked in to the black hole, while the other escapes.
When this happens, the particle that escapes -- whether it's matter, antimatter, or a photon -- has real, positive energy, and the black hole loses a corresponding amount of mass to make up for it. This type of radiation is known as Hawking radiation, and is (IMO) Stephen Hawking's greatest contribution to science, that he determined the existence, magnitude, and energy spectrum of this radiation.
This radiation is maddeningly cold; the black hole at the center of our Milky Way would emit Hawking radiation of a temperature measured in the femtoKelvin range, or a few times 10-15 Kelvin. But as a black hole evaporates and loses mass, that temperature increases. It may take a googol years or so for a black hole to evaporate completely, but when it does you get a brilliant flash of energy that's as powerful as any nuclear explosion here on Earth!
And that's how we can see black holes: practically through their gravity and their X-rays, and in theory through light from all parts of the spectrum from their accretion disks and super-low-energy light from Hawking radiation! Maybe someday, we'll even be sophisticated enough to detect it. In the meantime, know that despite their name, they're not so black after all!










Question Regarding the Hawking Radiation:- Around the Black Hole , the negative Mass particle enters the black hole where as the Positive Mass particle escapes as Hawkins radiation, effectively reducing the mass of the black hole. But also at the same time we have matter having positive mass entering the blackhole as well. Now only if the NET mass entering the blackhole is negative, will the mass of the blackhole reduce, otherwise it will always increase, as there is more positive mass in the universe than negative. Is this thought correct?
Thanks for the animation of the Sag A* stars: I think the first announcement that they'd plotted orbits of some of these stars was one of the most exciting things I'd heard in astronomy for years. I think up till then it was still just about possible to argue that black holes might be a purely theoretical construct. Granted perhaps the centres of other galaxies perhaps offered plenty of evidence for professional astronomers, but it was a bit indirect for laymen.
If there were no very heavy mass at the centre of the galaxy, gravitational force there would tend to zero, I suppose, and you'd get a very strange stellar dance.
Is it correct that the masses of the plotted stars can be inferred from their spectra, and so a handle got on the mass of the black hole?
Does anyone know what timescale the animation covers? I'd guess it's about a decade or so.
Ethan, theoretically what would happen if a black hole collided with an antimatter black hole of equal mass?
@VDGG
"Now only if the NET mass entering the blackhole is negative, will the mass of the blackhole reduce, otherwise it will always increase, as there is more positive mass in the universe than negative. Is this thought correct?"
- yes, you are correct. Some time ago I read that any black hole which is more than our solar mass, can never really evaporate through Hawking radiation, because it will keep feeding at a greater rate then it radiates hawking radiation. When all "matter" is gone, there is still CMB radiation to "eat". Only very small BH can emit faster than they get a chance to absorb. Because the smaller they are, the more "energetic" the radiation is.
@ Peter
there's a year counter in top left corner of the animation :)
Although since the CMB is reducing, that statement isn't true: eventually the net energy flow will be out of the black hole.
Then there's expansion of the universe: when the event horizon is retreating faster than light speed, your singularity is naked. And all bets are off!
@ DavidL
In theory... total and absolute DOOOOM!! :D
But IMO it's not even possible for 2 reasons mostly.
1. Because I don't see a way of getting enough antimatter in one place without it being annihilated by normal matter in the process.
2. As far as I know, most (if not all) antimatter was destroyed by matter in the earliest stages of Universe. There is no "free" antimatter in the universe. At least not in some usable amount. So i think it's impossible. For the same reasons we don't have antimatter asteroids or planets...
@ wow
that;s true. But I can't even comprehend the time till then. First all the matter.. all of it. Then black holes on their own. Swallowing one another... And then... ever so slowly... radiating away trillions and trillions of solar masses till eternity basically...
Ever watched Brian Cox's "Secrets of the Universe"? He gives the numbers in that for how long.
As Carl Sagan pointed out, though, even that hugely long time is still no nearer infinity than the first second of existence.
Ethan:
Photons are the force carriers for the EM force, so...the magnetic field must be associated with what's going on outside the event horizon, yes? It can't be associated with anything that's already passed the event horizon. Does it arise from the Hawking radiation, the charged particles orbiting the black hole, something I'm missing, or some combination of those three?
The event horizon is that geometrical surface from which no escape to infinity can be made.
This doesn't mean you can't escape to 1m before losing all energy and falling back in...
"When this happens, the particle that escapes — whether it’s matter, antimatter, or a photon — has real, positive energy, and the black hole loses a corresponding amount of mass to make up for it"
I get the radiation thing, but how (why ?) should the black hole lose mass ?
The borrowing of energy in the virtual pair has to be paid back.
Best just go "The Maths works, lets leave it at that" :-)
Phil,
Conservation of energy. If a black hole radiates energy in the form of Hawking radiation, the rest of the universe (the part outside the event horizon of the black hole) must gain energy. This gain in energy must be offset by a loss of energy. The only place for this loss to occur is inside the black hole.
Now, the physics of black holes states that the "black holes have no hair". That is, all black holes with the same mass are identical (this ignores charged and/or rotating black holes for simplicity). Since that's the case, the only way for a black hole to lose energy is for it to lose mass (E=mc^2).
@ Phil
one thing to note is that Hawking radiation isn't tested. I'm really hoping for microscopic black holes at CERN. If they get them to form, they should last only for a fraction of a second before they evaporate through Hawking radiation.
If that happens, and they do radiate, then we can say that it's real.
The whole issue with BH mass, surface, QM...is really complicated, not only in terms of math, but logicaly. It has to do with information theory and all sorts of things which can't be tested. Sussking and Hawking spend decades arguing, and I think the conclusion was they are both right... in a way. So for now... we have use what we have.
Sean,
Thanks for the answer :)
My problem is: if i understand correctly, the radiation only "seems" to originate from the black hole, since what we're speaking about is pair of (virtual) particles (let's say photons) "born" outside the black hole event horizon:
one photon is trapped inside the black hole, the other escapes from the gravity well and "radiates" from it.
As I understand the law of conservation of energy, your explanation would be true if a photon inside the event horizon actually made it out (well... i know it couldn't, but let's pretend).
What I really don't understand is how a pair of virtual particles that's not being part of the black hole could actually influence it other than by adding mass or energy to it (from the particle being trapped)
Dunno if it's clear, though :)
Peter Dugdale (#2): in the upper left corner of the animation is a label giving the year of each observation. You can watch the years change and figure out the duration of the animation.
It is not necessary to measure the masses of the stars in order to compute the mass of the black hole. When the central object is much, much more massive than the orbiting objects (as in our own Solar System), the orbital periods and radii alone yield the mass of the central object.
"Conservation of energy"
Actually, Sean, the original requirement was to stop the violation of the laws of thermodynamics. If a black hole didn't radiate, then it would be decreasing entropy as it takes in material.
Hawkin found that formulating the laws of thermodynamics on a black hole produced the fact that the entropy of a black hole is given by its surface area for the event horizon.
He then tried to work out how this could be so.
And that was Hawkin radiation.
Wait, wait, wait...let me get this straight.... You made a post about being able to see black holes, and your lead-in quote WASN'T:
"Well, the thing about a black hole - it's main distinguishing feature - is it's black. And the thing about space, the color of space, your basic space color - is it's black. So how are you supposed to see them?" -Holly, Red Dwarf
This may be the first thing you've written on here that I've *completely* failed to understand.
@ Phil
maybe this passage from wiki will help you.
"One of the pair falls into the black hole whilst the other escapes. In order to preserve total energy, the particle that fell into the black hole must have had a negative energy (with respect to an observer far away from the black hole). By this process, the black hole loses mass, and, to an outside observer, it would appear that the black hole has just emitted a particle."
Negative energy equaling negative mass. Thus by absorbing the negative particle, it actually looks like it's emmiting a positive particle and loosing mass.
@Wow
"then it would be decreasing entropy as it takes in material."
also information would be destroyed. That was another motivation.
@BenHead: "Empty" space isn't so empty. We detect the black hole through its effects on other stuff in the Universe: stars, gas, and even passing photons (i.e., gravitational lensing).
@Sinisa:
Thanks a lot ! Didn't know we could actually talk about a particle with negative energy... but then it all makes sense :)
Wow,
Thanks. I'm not a physicist, so it's not surprising that the real answer is more complex than my simplistic understanding of things. Serious question for the physicists, though. In my post above, I ignored what happens with charged and/or rotating black holes. If Hawking radiation occurs in a charged and/or rotating black hole, and that black hole absorbs a particle with negative energy, can that be manifested by a change in the angular momentum and/or charge of the black hole rather than the mass? Thanks in advance.
"every black hole that exists in the vicinity of other matter (which is practically all of them) is thought to have an accretion disk... the matter in it accelerates"
Now, I assume the matter in accretion discs is experiencing a spiral or rotational acceleration not a purely radial acceleration.
So my question are all observed black holes rotating black holes? Or is there some reason to believe that any black hole is non rotating?
and can we in an extended sense think of a whole galaxy (e.g. milky way) as a rotating accretion disk? e.g. do galaxies always rotate in the same direction as their supermassive black hole at their center?
Black holes can and do have angular momentum. And charge.
As people work out how to be smart at the maths (or we just throw a bigger computer at the problem), we can investigate how a black hole can be shaped.
And you can even get them spinning in a torus shape.
Making the singularity visible to the outside world is suspected to be possible by speeding up the spin of a black hole.
Actually, I find that advances can come from just considering "What's the *consequence* of this?" pleasing.
If, for example, there had been no possible way of giving a black hole entropy, then it would likely have been completely dropped in favour of keeping the second law working.
If the instantiation of entropy had not produced a physically plausible scenario that not only gave the system the correct entropy but also the right spectrum without needing special pleating, again, the black hole theories would likely have been rated lower and put back for something with more explicit accordance with the currently know science.
If the consequences of black holes or their radiation hadn't meshed with the classical mechanically known thermodynamic laws, these newcomers would have been shelved.
I find that heartening to think about.
@ Phil
I guess we are not talking about negative mass "per say". More in terms of math. Because when you have virtual pair production, you need to have 0 again when they "pop-out" of existance. So, mathematically you can say that one particle is +1 the other -1.
Benhead, if I would have gone for any other quote, it would have been:
"Since that’s the case, the only way for a black hole to lose energy is for it to lose mass (E=mc^2)."
Losing energy is precisely the same as losing mass, in this context (gravity). The rest mass of a black hole is irrelevant. E=mc^2 is an equivalence, not a conversion that only applies sometimes. They are and always will be the same quantity, just in different units.
As a daily reader of your blog, this is my favorite post yet. Keep up the fantastic work Ethan!
@ Peter Dugdale
In October 2012 the UCLA team led by Professor Grez using the Keck telescope identified a new star - S0-102 - orbiting Sagittarius A* every 11.5 years. She asserts that its orbital characteristics prove the existence of a supermassive black hole "beyond reasonable doubt.
This star doesn't appear in the animation shown above.
Analysis of the orbits of S0-102 and the star, S0-02, which has a 16 year orbit, will facilitate more precise calculation of the mass and location of the supermassive hole at the galactic centre and will provide an acid test of general relativity. Check out:
http://www.sciencedaily.com/releases/2012/10/121004141749.htm
There's a diagram of these observed orbits at:
http://en.wikipedia.org/wiki/S0-102
Wow
Thanks for those thoughts. Very nice.
On that virtual pair thing, is that another case of quantum entanglement? As it, it is always the negative one that falls into the hole, because the moment it fell into the hole it HAD to have been the negative one?
Feel a bit sheepish that I didn't spot the timescale top-left in the animation: thanks for those who pointed it out. I blame it either on tunnel vision caused by reading glasses, or the gorilla effect: the one that walks through the basketball game and nobody spots.
Also should have realised (thanks Michael Richmond) that the orbital period is independent of mass of a much smaller body: "all bodies fall equally fast" applies to orbits as well.
Thanks also to "irritable" for the links to the latest news: yet more icing on the cake!
@ nomuse
It's not always the negative one. It doesn't have to be. You could have pair production where both particles cross the horizon and end in singularity. Or both stay outside, and the next moment they disappear.
But actually what happens with Hawking radiation is much more complex. It has to do with the event horizon appearing as a thermal bath to an accelerating observer near the horizon. Then that state of let's call it "excited quantum vacuum" has to abide by certain principles, the math says there exists a thermal equlibrium and that the temperature is not infinite. Thus something must get out. The rest is trying to figure what and how mathematically works.
If it's really happening in nature is a different question all together.
As for entanglement, I would say you'll have to be more precise in your question. Particles are not entangled themselves... their properties are. And they needn't be all of properties. And entanglement on it's own doesn't mean anything. In a way, the pair definitely gets entangled with a black hole, since one particles goes in. But as far as any information, it matters not. And any observation you do on the second particle, breaks the entanglement. So I don't see the relevance.
Maybe I don't understand your question.
Nomuse and Sinisa
Well I think Nomuse's question makes sense.
First let me paraphrase or tell what question triggers in me.
"Physical insight into the (Hawking radiation) process may be gained by imagining that particle-antiparticle radiation is emitted from just beyond the event horizon. This radiation does not come directly from the black hole itself, but rather is a result of virtual particles being "boosted" by the black hole's gravitation into becoming real particles. A slightly more precise, but still much simplified, view of the process is that vacuum fluctuations cause a particle-antiparticle pair to appear close to the event horizon of a black hole. One of the pair falls into the black hole whilst the other escapes. In order to preserve total energy, the particle that fell into the black hole must have had a negative energy (with respect to an observer far away from the black hole). By this process, the black hole loses mass, and, to an outside observer, it would appear that the black hole has just emitted a particle. In another model, the process is a quantum tunneling effect, whereby particle-antiparticle pairs will form from the vacuum, and one will tunnel outside the event horizon." wikipedia
So it seems to me that Nomuse is asking about Hawking radiation process; his questions seem to be:
1) In the Hawking radiation process, must the "negative energy particle that falls into the black hole" always be an antiparticle?
2) Are the particle-antiparticle pair entangled in terms of their various quantum properties?
My SPECULATIVE answers are the following:
1 answer) We can not determine whether a particle escapes the black hole or an antiparticle. BECAUSE WE DON'T SEE THE PARTICLE THAT ESCAPES HERE AND THE ANTIPARTICLE THAT ESCAPES THERE. What we see is the radiation of annihilation of the antiparticle/particle real pair and this is the Hawking radiation.
2 answer) Yes the virtual pair that results in a real particle (escaping from the black hole) and a real antiparticle (bewing captured by the black hole) [or vis a versa] is an entangled pair for every entanglable property of this pair of particles. (e.g. if one is spin + the other is spin -, if one is charge +, the other is charge - and so on). However, this is just a theoretical entanglement; since we never are able to measure the property of any single escaping particle from a black hole. What we can see and only what we can see escaping from a black hole is black body radiation of Hawking radiation which is photons.
I'm not sure about these answers; so I defer to anyone who understands better than my amateur specualtion.
In addition to these 2 answers I have a third answer also speculative (or maybe incorrect or maybe correct, I'm not sure).
3 answer) In addition to photon black body radiation (e.g. Hawking radiation under discussion); there is a kind of black body radiation for every elementary particle and antiparticle at high enough temperatures. Hence it is possible to interprete Hawking radiation as not only a black body spectrum of photons; but also at sufficiently high temperatures (ie. at sufficiently high energies) a black body spectrum of every particle and every antiparticle.
Thus in this sense (3 answer) a black body spectrum of for example electrons (conforming to Hawking radiation) could in be calculated and in principle be measured. And for sure such electrons would be entangled with their counterpart postirons captured by the black hole. And that entanglement experiment could perhaps be done by a fearless astronaut who measured the spin of the particle outside the black hole and it entangled antiparticle (or vis a versa) after the astronaut was captured inside the event horizon of the black hole.
But of course success of this experiment or NOT would not be communicable to anyone outside of the event horizon of the black hole.
Once again, I defer to any experts who understand. Thanks
Answer: No.
Not after it's interacted, either one. Keeping entanglement is difficult.
But one "entanglement" is possible to keep: if this is a particle, then what went in is an antiparticle. If this is an antiparticle, then what went in is a particle.
Note that despite this "information" getting out, there's nothing unusual or useful about it.
@ OKThen
"What we see is the radiation of annihilation of the antiparticle/particle real pair and this is the Hawking radiation."
No no no. That is not Hawking radiation. You don't observe the radiation of annihilation.
Think of it like this.
case 1 - pair shows outside of black hole, annihilates outside of black hole - nothing happens.
case 2 - pair shows outside of black hole, drops into black hole, annihilates - nothing happens
case 3 - pair shows outside of black hole, one particle drops in, the other doesn't... - hawking radiation
But all of this has nothing to with entanglement. Pair production is "by default" an entangled state. Meaning you have particle/antiparticle pair. And whatever property you choose for one particle, it has to be "anti" on the second one. But this is nothing special or strange in terms of black holes or pair production.
And you can't say " is an entangled pair for every entanglable property of this pair of particles." Because you just can't say something like that in QM. You can only measure ONE property. And that measurment breaks the entanglement. You have no apriori knowledge of all the states of the system.
I don't really understand your part about every particle behaving like a black body... so I'll leave that alone, but I have to strongly disagree with what you wrote about Hawking radiation and entanglement, because from what I read, it just doesn't work that way.
p.s. and don't forget, since this is QM and not classical mechanics. We can't really talk about this pair or that pair. We can only talk in terms of probabilities. In other words, of ALL the pair productions that might happen, at least SOME may be such that one pair memeber enters BH and other doesn;t. This might be 1in10 or 1in100 billion. Important thing is there is at least some. And thus is a mechanism for radiating.
But I have to also say that I find hawking's theory a bit strained. There is no president for it. We are unable to create quantum foam in the laboratory. It's a theoretical possibility it exists, but it might be that it doesn't. We really really need quantum gravity in order to know what's going on.
SL,
Just to clarify for you, although there are a whole host of things we'd like to have quantum gravity for, Hawking Radiation isn't one of them. Quantum gravity effects are only important when gravitational fields get extremely strong, and believe it or not, they're not strong enough at a black hole's event horizon to matter. At least, not for any black holes massive enough to exist in our Universe. (Tiny, microgram black holes maybe, but solar mass and up? No way.)
You can surmise the existence of Hawking radiation from QM alone (plus an event horizon), which is the easiest way to explain it. But if you want to know things like the rate of radiation and the Temperature spectrum of what comes out, you need to do quantum field theory (QFT) in curved spacetime. The curvature of spacetime is given by GR, the behavior of the vacuum at/near the event horizon is given by QFT, and that's all you need for Hawking radiation.
QFT and GR are our most successful physics theories ever, and if they are right, then Hawking radiation with the properties described is inevitable. FYI, although this was mind-blowing when it was discovered in the 1970s, it is now (as of a decade ago, in my experience) so standard that graduate students do this calculation for themselves for fun in their third year of grad school or beyond.
QFT in curved spacetime is hard, mind you, and I only did the calculation in Schwarzschild spacetime, not Kerr, but that encapsulates the vast majority of what we expect to happen. I have no doubt that Hawking radiation is a necessary (and someday, a non-negligible) part of this Universe.
@Ethan
Thank you for adding further insight into the matter. I was perhaps under the impression there are more "maybe's" then there are.
Namely from wiki as far as quantum foam: " Ordinarily, quantum field theory does not deal with virtual particles of sufficient energy to curve spacetime significantly, so quantum foam is a speculative extension of these concepts which imagines the consequences of such high-energy virtual particles at very short distances and times."
p.s.
the reason why I mentioned quantum gravity is because in case of Hawking radiation, it's QFT in curved spacetime.
Wow
As always, thanks for that concise clarification. Especially your explanation on the difficulty of keeping particles entangled.
Everyone,
Assume everything else that I said is speculative unless clarified by someone. Oh and though I say speculative, it is (or was) my best understanding (or guess). But until I learn that a speculative idea of mine is confirmed or discredited by an expert; it is just a speculative idea. (i.e. a learning hypothesis)
And it's that curvature that (IIRC) is how you get your asymmetry going and have one partner freed from its virtual existence. An infinite plane would not give you any asymmetry. A planck-scale one would give you enormous asymmetry.
Wow
Thanks for that important asymmetry detail; which I did not understand. Nice.
"a brilliant flash of energy that’s as powerful as any nuclear explosion here on Earth"
This is what happens when a galaxy-size black hole boils down to a few grams or something? A terminal instability? So there at the End of Everything when the Milky Way black hole has eaten everything in the local group and cosmic expansion has carried everything else off to undetectability ... the MWBH evaporates for a few gadzillion eras so that the observable universe is filled with this extremely cold and I suppose extremely uniform Hawking radiation ... when suddenly ....
Am I on the right track here?
Black holes are artifacts of a bad theory and figments of human imagination. However, the existence of super massive objects that have a finite dimension I do not doubt.
Well, there's nothing like a well reasoned argument.
This really helped me have a better understanding of black holes. The explanition of the gravity being compared to Earth and New York help me understand that even thoug they are small the amount of gravity that pulls is extremely strong. I think it is very intresting how we can see black holes with X-ray emmisions and Hawking radiation dealing with protons.
Wooooo.Very nice...
Perhaps a simplistic question:
If no light escapes given the immense gravity in a BH, then with Hawking radiation the BH eventually loses mass and hence gravity. Light will then escape. Have we seen the "winking " on of prior black holes in the observable universe?