Semi-Competent Astrophotography and Sidereal Days

I'm teaching my "Brief History of Timekeeping" class again this term, and as always, I'm tweaking things a bit. This is one of our "Sophomore Research Seminar" courses, intended to introduce students to academic research, so it's not specifically a physics class, but I'm choosing to take the statements about research outside the student's field at face value, and thus will be requiring them to do final projects involving some empirical measurement of the natural world, that being the essence of scientific research. (Last time, I included that as an option, but nobody took it, so I ended up reading 17 library research papers...)

Of course, this presents some challenges, as the courses can't have prerequisites. And the students I have are not actually sophomores, but first-year students from our Scholars program. So finding appropriate research projects that they can carry out somewhat independently is a tricky business. So I've been trying some things out.

One of the key ideas in the history of timekeeping is the distinction between the "solar day," the time required for the Sun to return to the same position in the sky, and the "sidereal day," the time required for a distant star to return to the same position in the sky. Thanks to the motion of the Earth as it orbits the Sun (greatly exaggerated in the PowerPoint slide that's the "featured image" above, which is a cartoon and not remotely to scale, don't bug me about that), these are not identical-- the sidereal day is shorter than the average day by about four minutes.

This is actually a great thing in terms of driving science and technology. The difference means that the Sun appears to move relative to the stars-- that is, the first constellation visible just as the Sun sets is different at different times of year. This provides a convenient way of marking the passage of seasons from the patterns of visible stars at night, and drove no end of speculation as to what might drive this apparent motion, which fuelled a lot of early science. (It's also the origin for the stubbornly persistent pseudoscience of astrology; you win some, you lose some...)

One thing that occurred to me as a possible project was to document the difference between these lengths. Our time system is based on the solar day, so if you take pictures of the sky at the same time on different days, you should be able to see the difference. But how feasible is this?

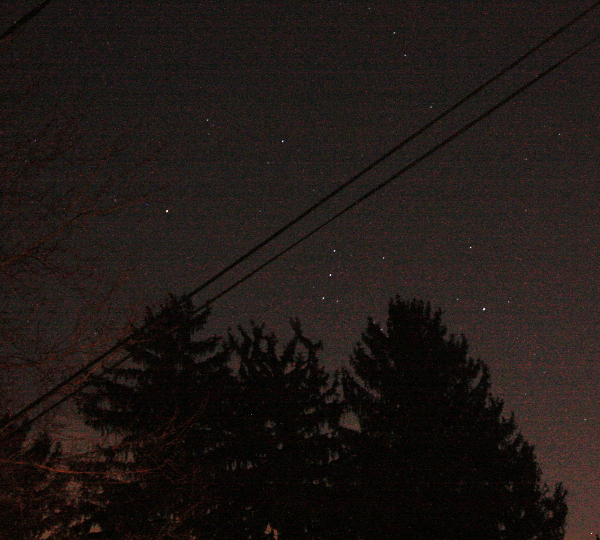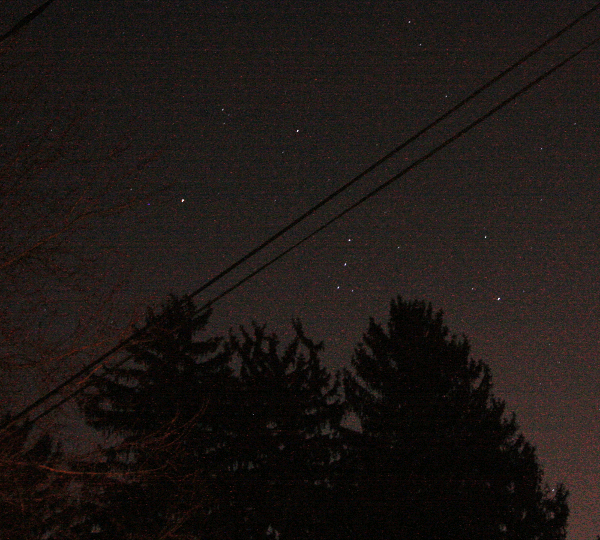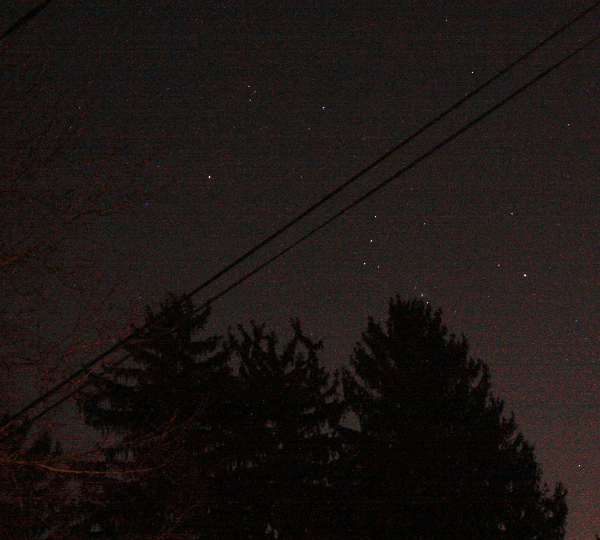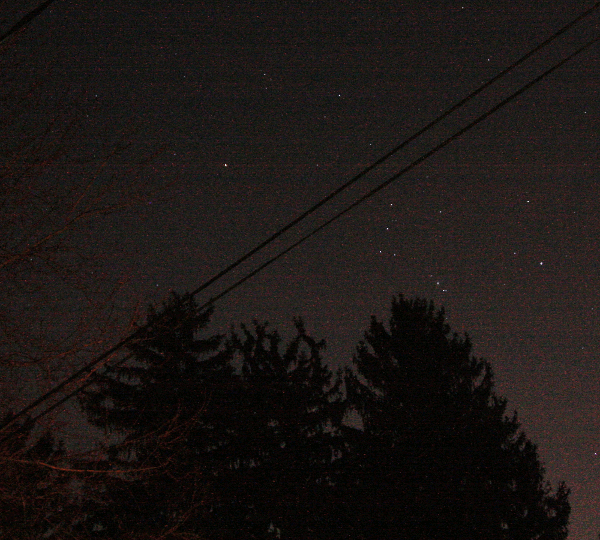
This week, on a whim, I decided to check this, using my DSLR camera and tripod on our back deck. We don't have a very clear view of the sky, but that ends up being a feature rather than a bug. I set the camera up on our patio table, and set it to do 2-second exposures with a wide-angle lens (10-18mm; because that's what happened to be on the camera when I started this), and took photos of a visible patch of sky at 4-minute intervals. I cropped these down and made the following animation of Orion rising over our back yard:

Orion rising over our backyard. Frames spaced by roughly 4 minutes.

The wide-angle lens and numerous obstacles in the yard actually ended up being a good thing when it came to making the animation-- I could use the branches of the big pine tree and the power lines that run across the frame to define a consistent crop region, and thus get a fairly stable field of view. (Shockingly, our aluminum patio table isn't the most stable mount you can get, particularly with me pacing back and forth to keep warm...)

So, what about the difference between solar and sidereal time? Well, for that, you need to repeat this on different days. The local climate didn't allow this observation on consecutive nights (read: it was cloudy Wednesday night), but I did manage to get pictures on both Tuesday and Thursday nights at the same time. Sadly, the Tuesday shots aren't very good-- they're all blurry, either because I just set the focus wrong, or because there was something on the lens, as it was right around zero Fahrenheit Tuesday night when I took these (I said this was done on a whim, not that it was a sensible whim...) Putting together two photos taken at the same time of day, two days apart, you can see that in addition to the focus issue, Orion is clearly in a different position relative to the trees in the later shot:

Two shots of Orion in early evening, two days apart, at the same time.

At four minutes difference between solar and sidereal days, a two-day interval ought to correspond to about eight minutes worth motion, so here's the same blurry shot together with a different in-focus shot, taken two days later but at a time eight minutes earlier than the first one:

Two shots of Orion in early evening, two days less eight minutes apart.

And I hope you'll agree that the stars in these two are in more or less the same positions relative to the trees, once you account for the focus issue.

If the Tuesday shots were less blurry, I could do a more precise calibration of the difference between these (you can look up the positions of the bright stars in Orion pretty easily, and use them to calibrate the angular scale of the image). A larger set of nights with clear images would let you do a pretty reasonable determination of the length of a sidereal day from this kind of thing.

Of course, like anything involving astronomy, this is highly dependent on getting good weather for several nights, and you need to make sure to take the photos at exactly the right time. But I think this is definitely feasible as a student project for anybody with a modest amount of experience with photography. Also, it's a good excuse for a blog post...